A Marxian Optimal Growth Model for Economies with Minimum Subsistence Wages

Abstract

This study constructs a two-sector two-class economic growth model to analyze an economy described in Marx’s Capital, where only the capitalist owns the means of production and maximizes the surplus value, while the worker provides labor in exchange for the minimum subsistence wage. Unlimited labor supply is the critical factor for the wage to be at the minimum subsistence level. In contrast to the Marxian optimal growth model, which indicates that the growth path in the capitalist economy follows a stable path to a steady state and is appropriate for analyzing the developed capitalist economy, our model demonstrates that, except in some rare cases, there is no stable path to a steady state in the economy and it is valid for analyzing an economy with an excessive labor supply. The results further indicate that it is common for capital to follow the process of unlimited self-growth, which causes the capitalist economy to be unstable.

Main article text

Introduction

By constructing the extended Reproduction Scheme, Marx analyzed the dynamic process of the capitalist economy with the minimum subsistence wage, a critical assumption in Marxian economics. In Marx’s assumption, the worker does not have assets or negotiating power in the labor market; therefore, it works under the minimum subsistence wage. Based on this condition, the capitalist can obtain a surplus value.

The Marxian optimal growth model constructed by Yamashita and Onishi (2002) is a modern version of the Reproduction Scheme, and the model summarized by Onishi (2011) discusses the social planner’s problem in an economy with two production sectors. The latter analyzes the interaction between capital and consumption goods sectors in Marx’s model by setting up a dynamic optimization problem. The social planner in the model optimally apportions the number of workers in both sectors by solving the social welfare maximization problem. Consequently, the model indicates a saddle-point path to the steady state. Additionally, the model interprets the steady state as the end of capitalism. Subsequently, Kanae (2013) presented a decentralized market economic model and provided the same path as the social planner model.

Based on the Reproduction Scheme, the Marxian optimal growth model is superior for considering roundabout production by dividing the production sector into two types. However, the decentralized model represented by Kanae (2013) significantly diverges from the traditional Marxian model in two ways. The first concern is the wage rate. The Marxian optimal growth model assumes that the marginal principle determines the wage rate; therefore, the rate is generally not in line with the minimum subsistence level. Second, the profit in the Marxian optimal growth model is assumed to be the difference between sales minus wages and capital costs, whereas the essence of profit, that is, the surplus value in Marxian economics, comes from the difference in sales minus the cost of hiring the worker. The root of these two is a fundamental assumption about whether workers can accumulate capital. Assuming that the worker can accumulate capital, the Marxian optimal growth model is plausible in an advanced society where the worker has the power to negotiate the wage rate and, therefore, is rich enough to save money. However, this is inappropriate for economies with an unlimited labor supply.

Owing to these differences, the decentralized model yields the same result as the social planner model, indicating a stable path through capitalism. However, Marx attempted to explain the instability of capitalism. Capitalists aim to maintain maximized profit and increase earnings by prioritizing investment in capital or wages, which results in increased productivity. By lowering wages to the minimum and cutting down on the workforce, the capitalist can save costs, enable the introduction of new technology, and have a more flexible response in times of economic depression. In the capitalist economy, which is the focus of Marx’s theory, unemployment and the struggle over income share were the norms, and, based on this, Marx concludes that capitalism is inherently unstable. However, regarding the Marxian optimal growth model and research that builds upon it, Tazoe (2011), Kanae (2013), and Li (2018) depict an economy with increasing wages, where the accumulation of assets has progressed, and this enables workers to accumulate assets. Therefore, those studies indicated that the growth path in the capitalist economy follows a stable path to a steady state.

In this study, faithful to Marx’s Capital, we construct a growth model with a minimum subsistence wage and demonstrate that the economy is unstable. The next section summarizes the characteristics of the economy in the early and later stages of economic development. Following this, we construct an optimal growth model with a minimum subsistence wage. Finally, in the last section, we compare our model with the Marxian optimal growth model to clarify the difference between the two models and how they explain the different stages of capitalism. The Marxian optimal growth model is appropriate for analyzing the developed capitalist economy, whereas our model is valid for analyzing an economy with an excessive labor supply.

Characteristics of the Early and Later Economic Development Stages

Lewis (1954) described a traditional economy in which the rural sector supplies workers in the urban sector at an unlimited rate. The wage difference between the two sectors is a critical factor for migration. Ranis and Fei (1961) described three phases of economic development based on this idea. In the first phase, the wage rate in the urban sector is always higher than that in the rural sector; therefore, workers migrate from rural to urban sectors. The excess labor supply in both sectors does not become zero because the urban sector’s wage rate cannot exceed the minimum subsistence level. Migration from the rural sector to the urban sector causes a shortage in the rural sector overall, and the wage difference becomes zero at equilibrium. ¹

In the first phase, workers in the rural sector move to the urban sector without an initial asset. They are hired at the minimum subsistence level under an excess supply of workers; therefore, they cannot accumulate their assets. The amount of capital stock is the only constraint on the capitalist optimization problem and determines the amount of labor employed.

The situation changes when the outflow of workers creates a shortage of workers in the rural sector. After the turning point, demand and supply mechanisms determine the wage rate in the urban and rural sectors.

The minimum subsistence level mainly determines the wage before the turning point, and after the turning point, the wage rises in line with labor productivity. Based on macroeconomic data describing the British economy from 1760 to 1913, Allen (2009) demonstrates that wages stagnated in the first half of the 19th century but began to grow in line with productivity after the middle of the 19th century.

Marx’s analysis focused on the British economy during the Industrial Revolution at the end of the 18th century and the beginning of the 19th century. During this period, there was a surplus of workers, and the wage determined by the capitalist who dominated the labor market only guaranteed subsistence. Therefore, Marx assumed the wage rate at the minimum subsistence level when he wrote, “the value of labor power is the value of the means of subsistence necessary for the maintenance of its owner” (Marx 1977, 678). Moreover, at the beginning of Economic and Philosophic Manuscripts of 1844, Marx indicated the determination of wage and wrote,

The lowest and the only necessary wage rate is that providing for the subsistence of the worker for the duration of his work and as much more as is necessary for him to support a family and for the race of laborers not to die. (Marx 1964, 3)

In summary, Marx analyzed the economic development stage, where the minimum subsistence mainly determined the wage, and the worker could not accumulate or own assets. Only the capitalist owns capital; therefore, capital transactions only occur among capitalists.

Neoclassical economics analyzes an economy close to the latter part of the 19th century, in which the labor market was competitive, and the marginal principle determined the wage rate. Consequently, wages were higher than the minimum subsistence level; therefore, workers could accumulate and rent assets to capitalists to obtain a return through the resource market.

Figures 1 and 2 simplify the economic activities of the capitalist and worker under the Marxian and neoclassical economics views. As Figure 1 illustrates, society has two economic subjects: capitalists and workers. According to the Marxist view, only capitalists monopolize the means of production and hire workers to take on production activities. However, workers who do not own any means of production provide labor to earn wage income and purchase consumption goods from capitalists.

Figure 1.

The Economic Activities of the Capitalist and Worker under the Marxian Economics View

Figure 2.

The Economic Activities of the Capitalist and Worker under the Neoclassical Economics View

In the neoclassical economics view, the worker is the owner of factors of production, and the capitalist is the user of factors of production. The capitalist buys or rents the factors of production to pay factor incomes which are wages for the labor supply and capital income for the asset. The capitalist will use factors of production to produce output in the way of goods and services, which the worker will purchase. In this way, the worker incurs their expenditures.

In conforming to the Marxist view, the following two assumptions are essential to analyze the economy in the early stages of economic development. First, we assume that the capitalist can determine the wage at the minimum subsistence level with an excess supply of workers. That is, the worker does not have negotiating power in the labor market and, therefore, works under the minimum subsistence wage. The minimum subsistence wage is one of the critical assumptions in Marxian economics, and there are many attempts to formalize Marxian theory by assuming the minimum subsistence wage. For example, Morishima (1973) and Roemer (1980) formulated a general equilibrium model with the minimum subsistence wage, and Uzawa (1964) analyzed the two-sector optimal growth model with a minimum subsistence wage. Second, the definition of profit is assumed to align with the surplus value. In Marxian economics, only the capitalist owns the means of production. Therefore, the surplus value equals the new value created by the worker in excess of their labor costs. That is, the surplus value equals revenue minus wage.

In our model, the capitalist, monopolizing the means of production, hires workers to undertake production activities. Furthermore, we assume the existence of two types of capitalists according to the final product they produce: the capitalist who produces the capital goods (Y ₁) and the capitalist who produces the consumption goods (Y ₂). Capitalists who produce consumption goods do not accumulate capital but purchase capital goods (K ₂) from capitalists who have capital goods every period, and this purchased capital depletes in one period.

Workers who do not own any means of production provide labor and obtain wage income in return. Using wage income, the worker purchases consumption goods from the capitalist firm. The total capital society held is K, and the total amount of labor is L which is assumed to be constant. Furthermore, we set the price of consumption goods to be unity and the price of capital goods to be P.

Let L ₁ and K ₁ be the quantities of labor and capital employed in the capital goods sector and let L ₂ and K ₂ be the quantities of labor and capital employed in the consumption goods sector, respectively. Consequently, we can represent the behavior of capitalists who produce capital goods, capitalists who produce consumption goods, and workers, as illustrated in Figure 3.

Figure 3.

Interaction of the Capitalist and the Worker

Model

In this section, we construct a model to analyze the economy, as summarized in Figure 3.

The Capitalist of the Capital Goods Sector

The production function of the capital goods sector is assumed to be,

(1) $$\begin{array}{c}{Y}_{1t}=A_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1},\end{array}$$

where Y _1t , L _1t , and K _1t represent the final production, labor, and capital inputs, respectively, at time t. Assuming that the function displays constant returns to scale, the equation α ₁ + β ₁ = 1 (here, 0 ≤ α ₁ ≤ 1, 0 ≤ β ₁ ≤ 1) is satisfied. The depreciation rate is δ₁. The profit (π _1t ) of the capitalist that produced capital goods is:

(2) $$\begin{array}{c}{\pi }_{1t}=P_t{A}_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-w_{1t}{L}_{1t}-P_t{\delta }_1{K}_{1t}.\end{array}$$

The capitalist profit maximization that produces capital goods is represented as follows:

(3) $$\begin{array}{c}\underset{w_{1t},L_{1t}}{\max }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\rho t}\left(P_t{A}_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-w_{1t}{L}_{1t}-P_t{\delta }_1{K}_{1t}\right)dt\end{array}$$

(4) $$\begin{array}{c}s.t.{\stackrel{.}{K}}_{1t}=A_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-{\delta }_1{K}_{1t}-K_{2t}\end{array}$$

(5) $$\begin{array}{c}{w}_{1t}\ge \underset{\_}{w}\end{array}$$

where ρ is the discount rate and w presents the minimum subsistence wage.

Assuming that the capital stock demanded by the consumption goods sector K _2t is given by the capitalist in the capital goods sector, we employ the following Hamiltonian:

(6) $$\begin{array}{c}\begin{array}{l}H=P_t{A}_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-w_{1t}{L}_{1t}-P_t{\delta }_1{K}_{1t}\\ +{\lambda }_t\left(A_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-{\delta }_1{K}_{1t}-K_{2t}\right)+{\mu }_t\left(w_{1t}-\underset{\_}{w}\right)\end{array}\end{array}$$

where λ_t and μ_t indicate Lagrange multipliers.

The first-order conditions of this optimization problem are as follows:

(7) $$\begin{array}{c}\frac{\partial H}{\partial L_{1t}}=0\to \left(P_t+{\lambda }_t\right){\beta }_1\frac{Y_{1t}}{L_{1t}}=w_{1t}\end{array}$$

(8) $$\begin{array}{c}\frac{\partial H}{\partial K_{1t}}=\rho {\lambda }_t-\stackrel{\cdot }{{\lambda }_t}\to \rho {\lambda }_t-\left(P_t+{\lambda }_t\right){\alpha }_1\frac{Y_{1t}}{K_{1t}}+{\delta }_1{\lambda }_t+P_t{\delta }_1=\stackrel{.}{{\lambda }_t}\end{array}$$

(9) $$\begin{array}{c}\frac{\partial H}{\partial {\lambda }_t}={\stackrel{.}{K}}_{1t}\to {\stackrel{.}{K}}_{1t}=A_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-{\delta }_1{K}_{1t}-K_{2t}\end{array}$$

(10) $$\begin{array}{c}\frac{\partial H}{\partial w_{1t}}=0\to -L_{1t}+{\mu }_{1t}=0\end{array}$$

(11) $$\begin{array}{c}\frac{\partial H}{\partial {\mu }_t}\ge 0\to {\mu }_t\left(w_{1t}-\underset{\_}{w}\right)\ge 0\end{array}$$

and

(12) $$\begin{array}{c}{\mu }_t^{*}\frac{\partial H}{\partial {\mu }_t}={\mu }_t^{*}\left(w_{1t}-\underset{\_}{w}\right)=0\to w_{1t}=\underset{\_}{w}\end{array}$$

where μ_t ^* indicates the Lagrange multipliers at the equilibrium.

Equation (11) implies that if μ _1t = 0, which satisfies the first-order condition,then from Equation (10), L _1t = 0, which indicates no employment in this economy. To obtain meaningful results, we focused only on the case where w _1t = w .

The Capitalist of the Consumption Goods Sector

The production function of the consumption goods sector is,

(13) $$\begin{array}{c}{Y}_{2t}=A_2{L}_{2t}^{{\beta }_2}{K}_{2t}^{{\alpha }_2}.\end{array}$$

The equation α ₂+ β ₂ = 1 (here, 0 ≤ α ₂ ≤ 1, 0 ≤ β ₂ ≤ 1) is satisfied, assuming that the function displays constant returns to scale. The profit maximization problem of the capitalist that produces consumption goods can be written as follows: ²

(14) $$\begin{array}{c}{\pi }_{2t}=A_2{L}_{2t}^{{\beta }_2}{K}_{2t}^{{\alpha }_2}-w_{2t}{L}_{2t}-P_t{K}_{2t}\end{array}$$

(15) $$\begin{array}{c}\underset{w_{2t},L_{2t}}{\max }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\rho t}\left(A_2{L}_{2t}^{{\beta }_2}{K}_{2t}^{{\alpha }_2}-w_{2t}{L}_{2t}-P_t{K}_{2t}\right)dt\end{array}$$

(16) $$\begin{array}{c}s.t.w_{2t}\ge \underset{\_}{w}.\end{array}$$

which can be down to the following static maximization problem:

(17) $$\begin{array}{c}\underset{w_{2t},L_{2t}}{\max }{A}_2{L}_{2t}^{{\beta }_2}{K}_{2t}^{{\alpha }_2}-w_{2t}{L}_{2t}-P_t{K}_{2t}\end{array}$$

(18) $$\begin{array}{c}s.t.w_{2t}>\underset{\_}{w}.\end{array}$$

The first-order conditions of this optimizing problem are as follows:

(19) $$\begin{array}{c}{L}_{2t}={\beta }_2\frac{Y_{2t}}{w_{2t}}\end{array}$$

(20) $$\begin{array}{c}{K}_{2t}={\alpha }_2\frac{Y_{2t}}{P_t}\end{array}$$

(21) $$\begin{array}{c}{w}_{2t}=\underset{\_}{w}\end{array}$$

The Worker

Under the Marxian economics in this article, the worker owns no means of production except for labor power. Moreover, the worker sells labor power and receives the necessary wage set at a minimum subsistence level by the capitalist.

The utility of the worker can be represented as:

(22) $$\begin{array}{c}\end{array}{U}_t=\{\begin{array}{ccc}U\left(c_t-\underset{\_}{c},\overline{l}-l_t\right)& if& c_t\ge \underset{\_}{c}\\ U_0& if& c_t<\underset{\_}{c}\end{array}$$

where l_t is the initial endowment of time and U ₀ is the constant.

A worker cannot survive if the amount of consumption c is lower than the minimum subsistence level c . In this situation, the worker’s utility is equal to U ₀.

In contrast, if consumption c is larger than the minimum subsistence boundary c , the worker’s utility increases with increasing consumption but with a diminishing increment rate. Utility also depends on the amount of leisure—the more leisure, the more utility, but with diminishing marginal utilities.

The worker’s maximization problem can be written as follows:

(23) $$\begin{array}{c}\max \underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\rho t}{U}_{t}dt\end{array}$$

(24) $$\begin{array}{c}s.t.{\stackrel{.}{B}}_t=r_t{B}_t+w_t{l}_t-c_t\end{array}$$

(25) $$\begin{array}{c}{c}_t>\underset{\_}{c}\end{array}$$

(26) $$\begin{array}{c}given{B}_0=0,w_t=\underset{\_}{w}\end{array}$$

where B_t and r_t are the number of assets and interest rate at time t. Initial asset B ₀ is assumed to be zero.

The first-order conditions of this optimizing problem are as follows: ³

(27) $$\begin{array}{c}{l}_t=\overline{l}s\end{array}$$

(28) $$\begin{array}{c}{c}_t=\underset{\_}{c}\overline{l}\end{array}$$

(29) $$\begin{array}{c}{\stackrel{.}{B}}_t=0\end{array}$$

The worker, who does not own any means of production in the initial period, is supposed to supply as much labor as possible and live under a minimum subsistence wage without any savings.

Market Equilibrium

This study focuses on urban areas where the surplus of labor is shifting from the agricultural sector. Unlimited labor supply allows capitalists to expand production under Cobb-Douglas technology by increasing labor demand without limitation. In such cases, capitalists can control their wages. The capitalist is supposed to set wages above or equal to a minimum subsistence wage to guarantee the survival of labor, although the capitalist can cut wages without a lower limit. It implies that:

(30) $$\begin{array}{c}{w}_{1t}=w_{2t}=w_t=\underset{\_}{w}\end{array}$$

is satisfied under the market equilibrium condition.

Moreover, labor is supposed to use up the wage for purchasing consumption goods as they are paid under a minimum subsistence wage. That is, the following equation holds under the market equilibrium condition.

(31) $$\begin{array}{c}{n}_t{c}_t=Y_{2t}=\underset{\_}{w}{L}_t\end{array}$$

where n_t is the number of workers hired at time t. L_t represents the number of workers required at the equilibrium condition.

Additionally, the following equations are satisfied in labor and capital markets under the market equilibrium condition:

In the labor market

(32) $$\begin{array}{c}{n}_t{l}_t=L_t=L_{1t}+L_{2t}\end{array}$$

In the capital market

(33) $$\begin{array}{c}{\stackrel{.}{K}}_{1t}=A_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-{\delta }_1{K}_{1t}-K_{2t}\end{array}$$

The price can be solved as

(34) $$\begin{array}{c}{P}_t=\frac{{\alpha }_2\underset{\_}{w}}{{\left(\frac{\underset{\_}{w}}{A_2}\right)}^{\frac{1}{{\alpha }_2}}{\left(\frac{1}{{\beta }_2}\right)}^{\frac{{\beta }_2}{{\alpha }_2}}}\end{array}$$

Instability of the Economy in the Marxian Economics

From the first-order conditions of the capitalist problem (7)(8)(9) and the conditions of market equilibrium (30)(31), we obtain the following equations:

(35) $$\begin{array}{c}\left(P_t+{\lambda }_t\right){\beta }_1\frac{Y_{1t}}{L_{1t}}=\underset{\_}{w}\end{array}$$

(36) $$\begin{array}{c}\rho {\lambda }_t-\left(P_t+{\lambda }_t\right){\alpha }_1\frac{Y_{1t}}{K_{1t}}+{\delta }_1{\lambda }_t+P_t{\delta }_1=\stackrel{.}{{\lambda }_t}\end{array}$$

(37) $$\begin{array}{c}\frac{{\stackrel{.}{K}}_{1t}}{L_t}=A_1{L}_{1t}^{{\beta }_1}{\frac{K_{1t}}{L_t}}^{{\alpha }_1}-{\delta }_1\frac{K_{1t}}{L_t}-\frac{{\alpha }_2\underset{\_}{w}}{P_t}\end{array}$$

Denoting

(38) $$\begin{array}{c}{k}_{1t}=\frac{K_{1t}}{L_t},(here,k_{1t}\ne 0)\end{array}$$

Equations (35), (36), and (37) can be rewritten as

(39) $$\begin{array}{c}{\alpha }_1\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}=A_1{\alpha }_2^{{\beta }_1}{k}_{1t}^{{\alpha }_1}\left(\frac{{\alpha }_1}{k_{1t}}+\frac{{\beta }_1\rho P}{{\alpha }_2\underset{\_}{w}}\right)-\left({\delta }_1+\rho \right)\end{array}$$

(40) $$\begin{array}{c}\frac{{\stackrel{.}{L}}_t}{L_t}=A_1{\alpha }_2^{{\beta }_1}{k}_{1t}^{-{\beta }_1}-{\delta }_1-\frac{{\alpha }_2\underset{\_}{w}}{P}{k}_{1t}^{-1}-\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}\end{array}$$

Substituting the value of k_t determined by Equation (39) into Equation (40), we can calculate the growth rate of the worker’s demand by the capitalist.

(39) $$\begin{array}{c}\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}=A_1{\alpha }_2^{{\beta }_1}{k}_{1t}^{{\alpha }_1}\left(\frac{1}{k_{1t}}+\frac{{\beta }_1\rho P}{{\alpha }_1{\alpha }_2\underset{\_}{w}}\right)-\left(\frac{{\delta }_1+\rho }{{\alpha }_1}\right)\end{array}$$

(40) $$\begin{array}{c}\frac{{\stackrel{.}{L}}_t}{L_t}=A_1{\alpha }_2^{{\beta }_1}{k}_{1t}^{-{\beta }_1}-{\delta }_1-{\left(\frac{\underset{\_}{w}}{A_2}\right)}^{\frac{1}{{\alpha }_2}}{\left(\frac{1}{{\beta }_2}\right)}^{\frac{{\beta }_2}{{\alpha }_2}}{k}_{1t}^{-1}-\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}\end{array}$$

Equations (39) and (40) describe the dynamics of k _1t and L_t , respectively.

Stability and the Existence of the Steady State

To discuss the stability of the model, we first solved Equation (39) graphically by dividing the right-hand side of the equation into two parts, X ₁, X ₂, where

$$X_1=\frac{A_1{\alpha }_2^{{\beta }_1}{k}_1^{{\alpha }_1}}{{\alpha }_1}\left(\frac{{\alpha }_1}{k_1}+\frac{{\beta }_1\rho P}{{\alpha }_2\underset{\_}{w}}\right)$$

and

$$X_2=\frac{{\delta }_1+\rho }{{\alpha }_1}$$

With $\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}$ on the vertical axis and k _1t on the horizontal axis, we draw the figure of Equation (39). The point(s) of the intersection of curves X ₁ and line X ₂ give a solution to the equation.

(41) $$\begin{array}{c}\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}=\frac{A_1{\alpha }_2^{{\beta }_1}{k}_1^{{\alpha }_1}}{{\alpha }_1}\left(\frac{{\alpha }_1}{k_1}+\frac{{\beta }_1\rho P}{{\alpha }_2\underset{\_}{w}}\right)-\left(\frac{{\delta }_1+\rho }{{\alpha }_1}\right)=0\left(here,k_{1t}\ne 0\right)\end{array}$$

Additionally, we can further discuss the steady state of the model based on the solution to Equation (40). Figures 4, 5, and 6 illustrate three possible cases.

Figure 4.

Case 1: Two Curves Intersect at Two Points

Figure 5.

Case 2: The Curves Intersect at One Point

Figure 6.

Case 3: No Interaction Between the Two Curves

Case 1: The two curves may intersect at two points, as illustrated in Figure 4.

There are two solutions, $k_{1t}^{*}$ , $k_{1t}^{**}$ , to Equation (41) in case 1. However, only the smaller one $k_{1t}=k_{1t}^{*}$ is stable. If k _1t is below $k_{1t}^{*}$ then $\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}>0$ , and the economy will accumulate capital in the capital goods sector. If the k _1t is above $k_{1t}^{*}$ then $\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}<0$ , the economy will decumulate capital in the capital goods sector. A steady state where $k_{1t}=k_{1t}^{*}$ exists in this case, and the economy will tend toward a steady state. However, if the k _1t is above $k_{1t}^{**}$ then ${\alpha }_1\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}>0$ , capital accumulates in the capital goods sector so that $k_{1t+1}>k_{1t}$ . This means the economy will accumulate capital toward infinity.

Case 2: The curves may intersect at only one point, as illustrated in Figure 5.

Case 2 represents an unstable model, even if there is a solution $k_{1t}^{*}$ in Equation (41). If k _1t is below $k_{1t}^{*}$ then ${\alpha }_1\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}>0$ , the economy will accumulate capital in the capital goods sector so that $k_{1t+1}>k_{1t}$ . And if the k _1t is above $k_{1t}^{*}$ then ${\alpha }_1\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}>0$ , capital accumulates in the capital goods sector so that $k_{1t+1}>k_{1t}$ . This means only the divergence path exists.

Case 3: The two curves may not meet at any point, as illustrated in Figure 6.

In this case, for all k _1t , ${\alpha }_1\frac{{\stackrel{.}{k}}_{1t}}{k_{1t}}>0$ , which also indicates that only the divergence path exists.

Simulation Results

Considering several sets of values for parameters close to the actual economy, Table 1 illustrates the simulation results of the growth path of capital in the capital goods sector and the number of workers required. According to Table 1, it is known that the capital goods sector’s capital productivity, that is, parameter α ₁, affects the growth path significantly.

Table 1.

Simulation Results

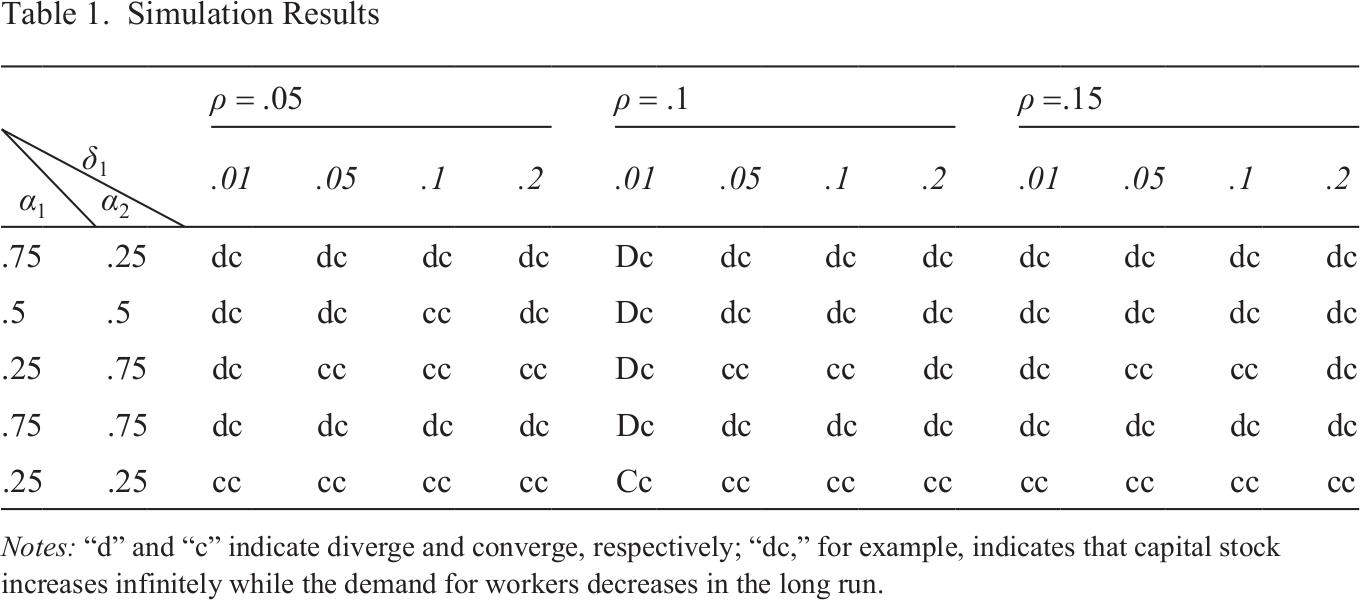
Notes: “d” and “c” indicate diverge and converge, respectively; “dc,” for example, indicates that capital stock increases infinitely while the demand for workers decreases in the long run.

Table 2.

Illustration of Differences Between Our Model and the Decentralized Model Presented by Kanae (2013)

A large value for parameter α ₁ represents high capital productivity. This brings a diverged growth path of capital in the capital goods sector and a converged growth path for the number of workers needed. High capital productivity in the capital goods sector indicates that capital is essential and capital accumulation is a priority for the economy. Capital accumulation increases infinitely, while unemployment increases because of the decreasing number of workers needed in such an economy, which is exactly the focus of Marx’s economy. However, a small value of parameter α ₁, representing low capital productivity, results in a converged growth path of capital in the capital goods sector and the number of workers required. This indicates a shrinking economy in which consumption takes precedence over capital investment; therefore, the economy shrinks along with increasing unemployment and decreasing capital investment.

The Decentralized Marxian Growth Model vs. Our Model

In the last section, we compare our model with the decentralized model extended by Kanae (2013) to clarify the distinction between them and the two different stages of capitalism to which the two models can be applied.

Figure 7 illustrates the basic setup of the model. Kanae (2013) ⁴ assumed that capitalists produce goods and pay wages and interest payments for capital input to workers. The capitalist maximization problem is expressed as follows:

The capitalist consumption goods sector

(42) $$\begin{array}{c}\underset{K_{1t},L_{1t}}{\max }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\rho }\left(P_t{A}_1{L}_{1t}^{{\beta }_1}{K}_{1t}^{{\alpha }_1}-w_{1t}{L}_{1t}-R_t{K}_{1t}\right)dt\end{array}$$

The capitalist capital goods sector

(43) $$\begin{array}{c}\underset{K_{2t},L_{2t}}{\max }\underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\rho }\left(A_2{L}_{2t}^{{\beta }_2}{K}_{2t}^{{\alpha }_2}-w_{2t}{L}_{2t}-R_t{K}_{2t}\right)dt\end{array}$$

where R_t is the rental payment for capital.

Figure 7.

Interaction of Capitalists and Workers in Kanae’s (2013) Model.

However, the worker is assumed to be an asset owner. The consumer provides labor and assets to the capitalist through the resource market and receives wages and interest income on assets in return. The consumer tries to maximize the overall utility under the budget constraint, which is expressed as:

(44) $$\begin{array}{c}\max \underset{0}{\overset{\infty }{{\displaystyle \int }}}{e}^{-\rho t}U\left(C_t\right)dt\end{array}$$

(45) $$\begin{array}{c}s.t.{\stackrel{.}{B}}_t=r_t{B}_t+w_t{l}_t-c_t\end{array}$$

Based on the above setup, Kanae (2013) provides the same result as the social planner, which indicates a stable path to a steady state, and capitalist economic growth follows that path.

We can confirm that the decentralized model presented in Kanae (2013) is much closer to the modern economic growth model while diverging from the traditional Marxian model.

However, this does not deny the importance of the optimal Marxian growth model. As mentioned above, there is a turning point in the process of economic development, and socioeconomic backgrounds vary widely before and after the turning point. Hence, building two different models to analyze the different stages is essential. Considering that only the capitalist owns the means of production, and the worker receives a minimum subsistence wage, our model is appropriate for analyzing the early stage of economic development. However, the Marxian optimal growth model is propitious to insight into the later stage of economic development because it assumes that the worker owns the assets and supplies capital to the capitalist through the financial market.

Conclusion

This study demonstrates the growth path of an economy in which only the capitalist owns capital and maximizes the difference (surplus value) between sales minus the cost of hiring workers (wage payments). The study concludes that such an economy has no stable path toward a steady state.

Considering the issues concerning Marx, the Marxian optimal growth model derives a stable path to a steady state under certain conditions, and capitalist economic growth follows that path. The Marxist optimal growth model depicts a harmonious society. By contrast, our model indicates that it is common for capital to follow the process of unlimited self-growth conceived by Marx.

However, this does not deny the importance of the optimal Marxian growth model. As mentioned above, there is a turning point in the process of economic development, and socioeconomic backgrounds vary widely before and after the turning point. Hence, building two different models to analyze the different stages is essential. Our model focuses on an economy with a constant minimum subsistence wage, described in Marx’s Capital, whereas the Marxian optimal growth model analyzes an economy with increasing wages, where the accumulation of assets has progressed and enables workers to accumulate assets.

Moreover, the Marxian optimal growth model, indicating that the growth path in the capitalist economy follows a stable path to a steady state, is appropriate for analyzing the developed capitalist economy. However, our model, demonstrating that there is no stable path to a steady state in the economy, is valid for analyzing an economy with an excessive labor supply.

Notes

1.

Following the analysis, Ranis and Fei (1961) and Harris and Todaro (1970) constructed a two-sector model to analyze the interaction between agricultural and nonagricultural sectors in the economic development process. Echevarria (1995, 1997), Gollin, Parente and Rogerson (2002, 2007), and Vollrath (2008) extended the analysis in the growth model framework.

2.

Here we assume that the capitalist shares the same time preference ρ.

3.

For the sake of simplicity, here we assume the same time preference for both the worker and the capitalist.

4.

Instead of using the terms “the capitalist” and “the worker,” Kanae (2013) adopts the terms “the firm” and “the household.”

References

1. R. C. Allen 2009. “Engels’ Pause: Technical Change, Capital Accumulation, and Inequality in the British Industrial Revolution.” Explorations in Economic History 46 (4): 418–435.

2. C. Echevarria 1995. “Agricultural Development vs. Industrialization: Effects of Trade.” Canadian Journal of Economics 28 (3): 631–647.

3. C. Echevarria 1997. “Changes in Sectoral Composition Associated with Economic Growth.” International Economic Review 38 (2): 431–452.

4. D. GollinS. ParenteR. Rogerson. 2002. “The Role of Agriculture in Development.” American Economic Review 92 (2): 160–164.

5. D. GollinS. ParenteR. Rogerson. 2007. “The Food Problem and the Evolution of International Income Levels.” Journal of Monetary Economics 54 (4): 1230–1255.

6. J. HarrisM. Todaro. 1970. “Migration, Unemployment and Development: A Two-Sector Analysis.” American Economic Review 60 (1): 126–142.

7. R. Kanae 2013. The Marxian Optimal Growth Theory. [In Japanese.] Kyoto: Kyoto University Press.

8. W. A. Lewis 1954. “Economic Development with Unlimited Supplies of Labour.” Manchester School 22: 139–191.

9. C. Li 2018. “China’s 2009–2050 Economic Growth: A New Projection Using the Marxian Optimal Growth Model.” World Review of Political Economy 9 (4): 429–450.

10. K. Marx 1964. The Economic and Philosophical Manuscripts of 1844. Edited by D. Struk, translated by M. Milligan. New York: International Publishers.

11. K. Marx 1977. Capital: A Critique of Political Economy, volume 1. Translated by B. Fowkes. New York: Penguin Books.

12. M. Morishima 1973. Marx’s Economics: A Dual Theory of Value and Growth. Cambridge University Press.

13. G. RanisJ. C. H. Fei. 1961. “A Theory of Economic Development.” American Economic Review 51: 533–565.

14. H. Onishi 2011. “The Marxian Optimal Growth Model, Reproduction Scheme, and General Law of Capitalist Accumulation.” World Review of Political Economy 2 (4): 603–634.

15. J. Roemer 1980. “A General Equilibrium Approach to Marxian Economics,” Econometrica 48 (2): 505–530.

16. A. Tazoe 2011. “Parameter Estimation for the Marxian Optimal Growth Model.” World Review of Political Economy 2 (4): 635–645.

17. H. Uzawa 1964. “Optimal Growth in a Two-Sector Model of Capital Accumulation.” The Review of Economic Studies 31 (1): 1–24.

18. D. Vollrath 2008. “How Important are Dual Economy Effects for Aggregate Productivity?” Journal of Development Economics 88 (2): 325–334.

19. Y. YamashitaH. Onishi. 2002. “Reconstructing Marxism as a Neoclassical Optimal Growth Model.” [In Japanese.] Study on Politics and Economy 78: 25–33.