The Formalization of Marx’s Economics : A Summary Attempt Taking as an Example the First Volume of Capital

Abstract

Whether Marx’s economics and Marxist political economy can be formalized using mathematics is a controversial and divisive issue. From studying the text of Capital and its manuscripts, it emerges that Marx’s research on the capitalist mode of production involves the application of mathematical methods. Marx creates a set of symbols related to the social production of capitalism, and on that basis constructs an economic model corresponding to his theoretical analysis. Obviously, the mathematical method is an integral part of Marx’s economic methodology. In a certain sense, using mathematical methods to study and formalize Marx’s economics is in line with Marx’s approach to economic topics. However, the formalization of Marx’s economics must strictly observe the relevant principles; otherwise, it will lead to serious problems.

Main article text

Introduction

Whether Marx’s economics and Marxist political economy can be formalized using mathematics is a controversial and divisive issue, especially in China’s academic field. Among the questions involved here is whether the mathematical method is among the research tools and narrative methods used in Marx’s economics. The author has previously written an article discussing this issue and reflecting on it (Zhang 2009). It remains necessary, however, to provide a further discussion of this issue based on the text of Marx’s economic works. The present article characterizes Marx’s economics using the example of Capital, since this is the most important of the works in which Marx systematically elaborated his economic theory.

Textual studies of Capital and its manuscripts show clearly that Marx, as he sought to reproduce the capitalist mode of production as a concrete object understood in theoretical terms, made extensive use of mathematical methods in his theoretical analysis and in his narrative method of proceeding from the abstract to the concrete. In Capital and its manuscripts, the capitalist economic categories obtained from theoretical analysis are used directly as economic variables, and the quantitative-structural characteristics of the economic categories are described using definite equations. These definite equations can often be converted into corresponding functional equations. At the same time, Marx expressed the economic relations between economic categories as functional relations, and described economic laws of a causal nature using functional equations so as to formalize them. In particular, Marx creates a set of symbols relating to the social production of capitalism, and on that basis constructs an economic model corresponding to his theoretical analysis. He formalized his theory using mathematics, which could be used as an analytical tool to derive and prove the economic laws of capitalism. In terms of their operational scope, mathematical methods were applied by Marx not only for analyzing the quantitative relations within the capitalist mode of production, but also for making a qualitative analysis of the intrinsic nature of the capitalist mode of production and its interrelationships. The mathematical methods Marx employed include constant mathematical methods, variable mathematical methods, game theory methods, and even stochastic mathematical methods.

Capital and its manuscripts reveal that Marx’s mathematical methods are not aimed at making a purely quantitative analysis of capitalist economic relations and of the process of reproduction. Rather, their real purpose is to carry out a theoretical analysis of capitalist economic relations and of the process of reproduction; to reveal the essential relations inherent in the capitalist mode of production; and to describe and reproduce these relations in mathematical form, that is, to express economic ideas through mathematics. What emerged from Marx’s use of mathematical methods is the mathematical approach to economics. Marx can be considered to have created an example of the use of mathematics at the abstract level through refined symbolic language combined with a system describing capitalist social production, and through the creation of a mathematical model system describing the capitalist mode of production. In this way, he brought the application of mathematics in political economy to a new height.

The reason why Marx had to use mathematical methods was determined by the objective nature of the capitalist mode of production. This reflects the qualitative and quantitative unity that exists in the capitalist mode of production as the object of study of Capital, as well as in the corresponding relations of production and exchange. The same qualitative and quantitative provisions and the same unity of quality and quantity are to be found in the laws of economic movement and economic categories of capitalist society, as revealed in Capital. For example, capital is a social production relationship based on wage labor, and the minimum amount of prepaid capital and wage labor determined by capital relations is a prerequisite for the formation of capital relations. If the amount of prepaid capital and wage labor is lower than this minimum, capital relations cannot be formed. Through mathematical derivations, Marx provided a general model of the minimum quantity required for the conversion of money into capital (Marx and Engels 2010, 311–314). For this reason, the analysis and theoretical reproduction of the capitalist mode of production cannot be achieved without the application of mathematical methods based on historical materialism, dialectical materialism, and scientific abstraction. It is obvious that the mathematical method is an important part of Marx’s methodological system of economics, though within this system the mathematical method and its technicalities are in a subordinate position.

Therefore, using mathematical methods to study and restate Marx’s economics, while formalizing and making explicit the methods involved, corresponds in a certain sense to the heuristics of Marx’s economics, and is an important aspect of adherence to his economics. To summarize, there are two cases in which Marx applies mathematical methods. In the first, he provides mathematical models and mathematical equations directly, by using his refined symbolic language of capitalist social production. This symbolic language runs through the three volumes of Capital, especially the third part of the second volume.

The second case involves providing theoretical generalizations or arguments that imply formalization by expressing mathematical relationships in natural language. In this sense, many laws and principles of Marx’s economics are able to be formalized and can be manifested in Marx’s symbolic language. Due to limitations of length, this second case of Marx’s mathematical methods will only be revealed in a summary manner, focusing on the first volume of Capital as an example.

The Formalized Outline of the First Volume of Capital

The first volume of Capital, consisting of seven parts and 25 chapters, studies the production process of capital. The content of this volume can be summarized using three basic theories: the labor theory of value in a narrow sense (part 1), the theory of the production of surplus value (parts 2–6), and the theory of capital accumulation or surplus value capitalization (part 7). It should be noted that the labor theory of value runs through all of Capital. Part 1 of the first volume sets out only the most basic content of the labor theory of value. This basic content will be referred to continuously as the development of the theory proceeds. The present text will examine the discussion of the labor theory of value in the third volume of Capital, in the scope that is required.

The Formalized Outline of the Labor Theory of Value in a Narrow Sense

Marx’s study of the capitalist mode of production begins with his analysis of the commodity. The commodity is the expression of the social wealth that dominates the capitalist mode of production, and the individual commodity is the elemental form of this wealth. Let $W^{n+1}$ be the set of commodities or commodity space that expresses capitalist wealth. In this scheme, $W^n=(w_1,\dots ,w_n)$ is a subset of the set of commodities, and w_i corresponds to the elements that make up the subset of commodities. The formalized expression of Marx’s starting point for his study of the capitalist mode of production is then: ¹ $w\in W^n\subset W^{n+1}.$

The commodity that provides the starting point for the study of Capital is a theoretical abstract commodity. It is a commodity that abstracts away capital relations when the capitalist mode of production is taken as a premise. This abstraction is, however, consistent with the historical development of commodity production.

In his analysis of commodities, Marx starts with their exchange value, and reveals the commodity value that lies hidden behind the exchange value that is formed by the condensation of general labor. In this way, he establishes an intrinsic link between the value of commodities and the general human labor consumption. The exchange value of a commodity can be expressed as: $x{w}_a=y{w}_b\Rightarrow w_a=\frac{y}{x}{w}_b.$

The general human labor consumption is the result of the labor input of the commodity producer A. The link between commodity value and general human labor consumption is then expressed as the relationship between labor input and value output, i.e., $f:A\to w.$ With regard to the nature of the labor that forms commodity value, it should be noted that commodity value is determined not by the individual labor consumption but by the socially necessary labor consumption, i.e., the socially necessary labor time. Socially necessary labor time depends on the normal production condition p_c , the average social proficiency l_a , and labor intensity ${\overline{l}}_h.$ Under these conditions, the socially necessary labor time for the production of a unit of a commodity is:

$$t_s=\overline{t}=\frac{{\displaystyle \sum }_{i=1}^n{t}_i}{Q}\mathrm{or}{t}_s=Et={\displaystyle \sum }_{j=1}^n{b}_j{t}_j.$$

The latter is a form of mathematical expectations, where b_j = q_j / Q, ${{\displaystyle \sum }}^{}{b}_j=1.$ Socially necessary labor time in another sense, as proposed by Marx in the third volume of Capital, can be expressed as $T_s=t_s{q}_D,$ that is, the total amount of necessary labor consumed by society to produce a certain amount of goods (q_D ) for which there is a demand and the ability to pay. If the commodity supply formed by decentralized decision producers is greater than the demand with the ability to pay, that is, if $q_S>q_D,$ then even if the unit commodity contains only the socially necessary labor time t_s the amount of labor consumed by the total amount of goods still exceeds the amount of socially necessary labor, and the unit commodity can only be sold at a price lower than its value, i.e.,

$$t_s{q}_S(1+r)>T_s,\mathrm{hence}\frac{T_s}{q_S(1+r)}=t<t_s,p=t=t_s(1-\frac{r}{1+r}),$$

where p is price. The labor cost per unit of commodity with $t_s\frac{r}{1+r}$ cannot be realized, and the producer’s labor cost cannot be compensated by value. In some literature, the price that deviates from commodity value is regarded as that determined by socially necessary labor time in the second sense. This view is clearly not valid because it denies the deviation of price from value. Another sense of socially necessary labor time is determined on the basis of the value per unit of the commodity, i.e., the determination of value as discussed in the first volume of Capital, and then taking into account the scale of the social demand with the ability to pay. This is the total amount of socially necessary labor time for the production of a certain commodity, which cannot determine the value per unit of the commodity.

If Marx’s analysis in the third volume of Capital of the market value formed by intrasectoral competition is taken into account, the market value can be expressed as follows:

$$t_s=\overline{t}=\frac{q_g{t}_g+q_m{t}_m+q_b{t}_b}{q_g+q_m+q_b},$$

where the subscripts g, m, and b denote superior, medium, and inferior production conditions, respectively, t_i (i = g, m, b) is the individual labor time for producing similar goods per unit of commodity, q_i is the output under different conditions, and $\overline{t}$ is the social average labor consumption. That is, under the given conditions, the socially necessary labor time takes the form of average labor time consumption. If $\overline{f}\in F$ is the average production condition of the sector, the market value can be simultaneously expressed as:

$$\{\begin{array}{c}{t}_s=t_i\\ s.t.f_i=\overline{f}\in F;\frac{q_i}{{{{\displaystyle \sum }}^{}}_{i=g}^b{q}_i}>>\frac{{{{\displaystyle \sum }}^{}}_{i=g}^b{q}_i-q_i}{{{{\displaystyle \sum }}^{}}_{i=g}^b{q}_i}\end{array}$$

If the social demand with the ability to pay for a certain commodity is $Q={\displaystyle \sum }_{i=g}^b{q}_i,$ there will be three possible cases of value determination:

when $Q=D=q_g=S,$ the market value is determined by the superior condition, i.e., $t_s=t_g$ ;

when $D>>q_g=S,$ and $(D-a)=q_g+q_m=S,$ the market value is determined by the medium condition, i.e., $t_s=t_m$ ;

and when $(D-a)>>q_g+q_m=S,$ and $(D-\alpha -\beta )=q_g+q_m+q_b=S,$ the market value is determined by the inferior condition, i.e., $t_s=t_b.$ These conditions, once determined, are central to market price fluctuations and act as a regulator in short-term supply and demand.

The earlier-mentioned mapping $f:A\to w$ can be expressed as a function $w=f(A).$ This can be regarded as Marx’s value function or value production function, and its quantifiable form is $w=f(A)+\epsilon ,$ i.e., $w=E(w|A_i)+w_u.$ Here w_u is the deviation from the statistically significant mean value of value $E(w|A_i)$ , which is also created by living labor. Considering the complexity of labor, the value function can be expressed as $w=h(t)f(A),$ where h(t) is the labor complexity coefficient that varies with time.

Marx made a comprehensive analysis of the factors that determine labor productivity, and established an intrinsic link between these factors and output Q. This is the labor productivity function given by Marx:

$$Q=f(\overline{A},S_p,P_c,P_m^s,N),\mathrm{and}\frac{\partial Q}{\partial f}>0,$$

where $\overline{A},S_p,P_c,P_m^s,andN$ are the average proficiency of workers, the degree of scientific development and its application to technologies, the social integration of production processes, the size and effectiveness of the means of production, and natural conditions, respectively. This productivity function is obviously different from and superior to the popular neoclassical production function. Based on the nature of value determination, Marx derived the law that the amount of commodity value is inversely proportional to labor productivity. In this article, the law is defined as a fundamental theorem of the labor theory of value, which can be formalized as follows:

$$W=f(t_s,Q)\mathrm{and}\frac{\partial W}{\partial t_s}>0,\frac{\partial W}{\partial Q}<0.$$

That is,

$$W=\frac{t_s}{Q},dW=\frac{\partial W}{\partial t_s}d{t}_s+\frac{\partial W}{\partial Q}dQ=\frac{1}{Q}d{t}_s-\frac{t_s}{Q^2}dQ,$$

where Q is the labor productivity expressed as output, or labor productivity (L) measured by output Q per unit of time, t can be expressed as:

$$W=f(L,t_s)\mathrm{and}\frac{\partial W}{\partial L}<0,\frac{\partial W}{\partial t_s}>0$$

$$\{\begin{array}{c}L=\frac{Q}{t}\\ w=t_s\end{array}$$

Obviously,

$$\begin{array}{l}{t}_s=\alpha t_i,\mathrm{if}{t}_i>t_s,\mathrm{then}\alpha <1;\mathrm{if}{t}_i<t_s,\mathrm{then}\alpha >1,\\ \frac{\alpha t_i}{Q}=\frac{1}{L},w_i=\frac{\alpha t_i}{Q},\frac{d{w}_i}{d(\alpha t_i)}>0,\frac{d{w}_i}{dQ}<0,\end{array}$$

or

$$\frac{t_s}{Q}=\frac{1}{L},w=\frac{t_s}{Q},\frac{dw}{d{t}_s}>0,\frac{dw}{dQ}<0,$$

where L is labor productivity, t_i is individual labor consumption, and α is the coefficient of the conversion of individual labor consumption into socially necessary labor consumption.

The fundamental theorem of the labor theory of value shows that the value per unit of a commodity and the total value of the commodity are both inversely proportional to labor productivity. To illustrate this, it is assumed that labor productivity is $l_0=q_0$ at the beginning of the period $t=0$ and the value per unit of the commodity is $w_0=\frac{t_s}{q_0}$ . If the total amount of commodities needed by society is n, then the total value amount of the commodity is $n{w}_0=n\frac{t_s}{q_0}=W_0$ . If it is then assumed that time t = 1, labor productivity increases from q ₀ to $q_1=q_0(1+r),$ and the value per unit of commodity w ₁ will decrease to $w_1=\frac{t_s}{q_0(1+r)}<w_0$ . If we now examine the socially necessary labor time nw ₁ required to produce the same total amount of commodities n, then we have $n{w}_1=n\frac{t_s}{q_0(1+r)}=W_1$ . Obviously, if $W_1<W_0$ , the total amount of socially necessary labor time will decrease by $W_0[1-{(1+r)}^{-1}]$ , which is the total labor time saved in the whole society on account of the increase in labor productivity. This is a figure of great significance. If we examine the same labor time, i.e., constant labor time, the total amount of commodities produced in the same labor time after the increase in labor productivity is necessarily greater than before, that is, $q_0(1+r)>q_0$ . It is obvious that the value per unit of the commodity and the total value of the commodity are both inversely proportional to labor productivity. ²

The contradiction between socially necessary labor time and individual labor time is the main way in which the law of value regulates the production and circulation of commodities. The analysis of this contradiction and of its role runs through the whole process of Marx’s analysis of the capitalist economy and reveals the endogenous nature of competition, technological progress, producer differentiation, and economic instability in commodity production and capitalist production. Marx’s analysis can be described as follows.

The relationship between individual labor consumption and socially necessary labor consumption can be expressed as $t_s=\alpha t_i$ . If $t_i>t_s$ , then $\alpha <1$ ; if $t_i<t_s$ , then $\alpha >1.$ Set $\frac{\Delta R(t)}{\Delta t}=\eta (t_s-t_i)$ :

If $t_i<t_s$ , then $\frac{\Delta R(t)}{\Delta t}>0$ ; if $t_i>t_s$ , then $\frac{\Delta R(t)}{\Delta t}<0$ .

In order to obtain excess returns ΔR, producers will compete to adopt advanced technologies to increase labor productivity and reduce individual labor consumption ( $t_i<t_s$ ). The new technology becomes the determining condition of socially necessary labor time as soon as the competition among producers for this purpose makes it widespread and it becomes the determining condition of production, i.e., when among all producers N the number of producers n who adopt the new technology becomes a majority, and $n>N-n$ . Under the new conditions, excess returns disappear, the value per unit of the commodity decreases, there is a general advance in technology, productivity rises, and output increases. Producers must further improve technology and increase labor productivity if they want to achieve excess returns. It is this dynamic process that continues to drive technological progress (see Figure 1 in which $t_i<t_s$ is represented by $t_j<t_s$ ).

Figure 1.

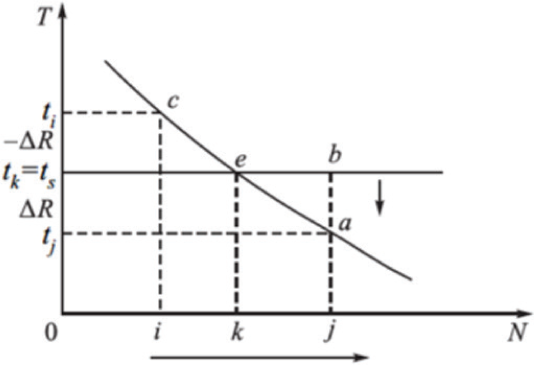
Labor Productivity and the Value of Commodities

Notes: The horizontal axis denotes Producer N, the vertical axis denotes Labor Time T, and the downward sloping curve denotes individual labor time corresponding to producer.

Marx in his analysis pointed out that the competition of commodity producers for excess returns, determined by the contradiction between individual labor consumption and socially necessary labor consumption, was a “prisoner’s dilemma” or a conflict between individual rationality and collective rationality of commodity producers. The above competitive process can be described as a static game process with full information from the perspective of producer behavior.

Let there be two types of producers A_i and A_-i who have the same set of strategies S: improving technology and increasing labor productivity φ; or keeping technology and productivity constant µ, i.e., $S_{i,-i}=\{\phi ,\mu \}$ :

$$S=\{(\mu ,\mu ),(\mu ,\phi ),(\phi ,\mu ),(\phi ,\phi )\}.$$

The corresponding set of payments $u_{i,-i}(S)$ is then:

$$U=\{(w,w),(w-\Delta R,w+\Delta R),(w+\Delta R,w-\Delta R),(w-r,w-r)\}$$

and $w+\Delta R>w>w-r>w-\Delta R$ . Obviously, for both types of producers, φ is a strictly dominant strategy, $s_{i,-i}=(\phi ,\phi )$ is the optimal strategy combination, and $u_{i,-i}=(w-r,w-r)$ is the payment. The results of competition include the disappearance of excess return ΔR, a decline in the value of commodities from w to $w-r$ , and the spread of new technologies, along with their becoming a new determinant of socially necessary labor time (Zhang and Xue 2020).

The process of competition, determined by the contradiction between socially necessary labor and individual labor, is an important mechanism of technological progress, of relative surplus value production, of an increase in the organic composition of capital, and of the tendency of the average rate of profit to fall. At the same time, it is also the theoretical basis for understanding these phenomena. Competition is an important phenomenon in the study of economics, and Marx’s economics has provided a complete and scientific description of it.

Marx’s analysis of the contradiction between socially necessary labor time and individual labor time allows a series of economic and social consequences resulting from this contradiction to be further expressed, including the differentiation of producers. If the individual labor consumption of producers is of the type $t_i>t_s,$ the labor consumption will not be compensated by social standards and producers will be threatened with bankruptcy, which in turn will lead to differentiation among producers. The differentiation process of producers can be described on a probability basis as:

$$\{\begin{array}{l}N=N_g+N_d+N_b=N(P({\overline{t}}_a)+P(t_a)P(\overline{b}|t_a)+P(t_a)(b|t_a))\hfill \\ {{\displaystyle \sum }}^{}P(\cdot )=1\hfill \\ \forall t_a\in (t_x>t_s)\hfill \end{array}$$

That is, the law of value regulates total labor distribution through the contradiction between socially necessary labor and individual labor, and divides all producers N into normal reproduction N_g , reproduction difficulty N_d , and bankruptcy N_b in terms of probability $P({\overline{t}}_a)$ and conditional probability $P(t_a)P(\overline{b}|t_a)$ and $P(t_a)(b|t_a).$ Contrary to what neoclassical economics holds, the process and result of market regulation are not in a non-differentiated steady state.

Marx’s social conditions for the production of commodities, i.e., the system of private division of labor or of social relations in which the parties treat each other as outsiders, can be expressed as:

$$\{\begin{array}{c}Q={(1-A)}^{-1}Y\\ E(q)=\{y|y\in Q,\rho y\le q^s\}\end{array}$$

Here Q is the division of labor, the total output vector E(q) is a social relationship that treats others as outsiders, Y is the final product vector, and the {.} part is the private right expressed by output as supply. It is only under such conditions that general labor acquires economic significance with value. Marx points out that the exchange of commodities begins first at the boundaries of communities, at their points of contact with other similar communities or with members of the latter. The argument is not that public ownership is the social basis of commodity exchange, but that different communities or members of different communities initially treat each other as outsiders, and recognize each other as private owners of the things to be exchanged. In other words, the members of these different or distinct communities relate to each other as independent outsiders, i.e., private owners.

In its analysis of the quantity specification of relative forms of value, Marx’s exposition features four cases. According to his derivations and conclusions, the first case, for example, can be expressed mathematically as follows.

Assuming $\frac{d{W}_a}{dt}\ne 0,\frac{d{Q}_a}{dt}=0,\frac{d{W}_b}{dt}=0;$ if $\frac{d{L}_a}{dt}<0,$ then $\frac{d{W}_a}{dt}>0,$ and the quantity of exchange (magnitude of relative value) Q_b increases, i.e., $\frac{d{W}_a}{d{Q}_b}>0.$

Assuming that $\frac{d{L}_a}{dt}>0,\mathrm{then}\frac{d{W}_a}{dt}<0,$ and the quantity of exchange (magnitude of relative value) Q_b decreases, i.e., $\frac{d{W}_a}{d{Q}_b}<0.$

Therefore,

$$Q_b=f(W_a)\mathrm{and}\frac{d{Q}_b}{d{W}_a}>0.$$

Combining the four cases, $Q_b=\frac{t_{s,a}}{t_{s,b}}$ can ultimately be obtained. In other words, the exchange ratio of a commodity or the magnitude of relative value of a commodity expressed in its equivalent depends on the proportion of necessary labor consumption condensed in the commodity. In the equation, Q is the magnitude of use value. According to Morishima, the conclusion reached by Marx cannot be applied because Marx did not see the problem of deciding the magnitude of relative value as a problem between industrial sectors. However, Morishima goes on to maintain that Marx’s first rule can be applied only if there are technological advances in the wage goods or luxury goods industries (Morishima 2017, 79). Morishima has apparently failed to notice that the third and fourth cases summarized by Marx include the impact of technological progress in the capital goods industry on the magnitude of the relative value of commodities.

If we let $W^{n+1}$ be the commodity space or set of commodities, W ₀ is the commodity that plays the role of an equivalent in $W^{n+1}$ and W ₀ will become the material expression of the value of W ⁿ Marx’s view that the general form of value is a socially recognized form can be expressed as $W^n=W_0.$

In his analysis of currency or commodity circulation, Marx proved that the possibility of crisis existed even under simple commodity conditions by analyzing the difficulties of changing the first form of the commodity and the function of money as a means of payment. According to Marx, this possibility of crisis can be attributed to the fact that the inherent contradictions of commodity production make the process of commodity realization uncertain. In other words, change in the first form of the commodity is random. The process may be realized on a basis of no less than value (S ₁) or less than value (S ₂), or else it may not be able to be realized at all (S ₃) The probability distribution can be expressed as:

$$P(S=s_i)=p_i(i=1,2,\dots ).$$

It can also be expressed as a distribution function $F(s)=P(S\le s).$ The general realization difficulty or crisis for $S>D$ will be developed once p2 or p3 occurs with high probability. The six factors that lead to difficulty in changing the initial form of commodities, as pointed out by Marx’s analysis of both use value and value, are of a random nature. They can be expressed by the above probability.

The interruption of the means of payment function also results from the difficulty of changing the initial form of commodities. It is assumed that the maturity yield a debtor expects is $E(R_{t+n}),$ and $E(R_{t+n})\ge B{e}^{rt}$ (the principal of the debt plus interest). Nevertheless, what the debtor faces at maturity will be an uncertain return with the distribution $F(s)=P(S\le s).$ If p ₂ or p ₃ applies, the debt will not be paid off and a debt crisis may thus arise.

The law of currency circulation deduced by Marx can be described in a formalized way. While $G^d=f(P,V)$ is the circulation function, i.e., the demand for money function, and the price $P={\displaystyle \sum }_{i=1}^n{p}_i{q}_i,$ V is the velocity of currency circulation. Assuming $\frac{dV}{dt}=0,$ the circulation function is simplified to $G^d=P=f(W,W_g),$ where W_g is the value of the precious metal.

$$\begin{array}{l}\mathrm{If}\frac{dW}{dt}=0,\mathrm{then}\frac{d{G}^d}{d{W}_g}<0\mathrm{and}\mathrm{thus}\frac{dP}{d{W}_g}<0.\\ \mathrm{If}\frac{d{p}_i}{dt}=0,\frac{d{W}_g}{dt}=0,\mathrm{but}\frac{d{q}_i}{dt}\ne 0,\mathrm{then}\frac{d{p}_i}{d{q}_i}>0,\frac{d{G}^d}{d{p}_i}>0\mathrm{and}\mathrm{thus}\frac{d{G}^d}{d{q}_i}>0.\\ \mathrm{If}\frac{d{q}_i}{dt}=0,\frac{d{W}_g}{dt}=0\mathrm{and}\frac{dP}{d{p}_i}>0,\mathrm{thus}\frac{d{G}^d}{d{p}_i}>0.\end{array}$$

From $thescalarproduct<p,q>=P={\displaystyle \sum }_{i=1}^n{p}_i{q}_i$ , it follows that $P=p_1{q}_1+\dots +p_k(1\pm \pi )q_k+\dots +p_n{q}_n$ . Therefore $P=f(p_k)$ , where q_i is the quantity of the commodity i and G^d is the demand for the precious metal or money.

Assuming $\frac{dP}{dt}=0,$ the circulation function is $G^d=f(V)$ , i.e., $\frac{d{G}^d}{dV}<0.$ The law of currency circulation $\frac{P}{V}=G^d$ can be obtained from the above analysis. Where both P and V are variable, the amount of money in circulation depends on their change of direction and speed.

The Formalized Outline of Surplus Value Production Theory

Marx’s analysis of the capitalist mode of production begins with the contradiction inherent in the general formula for capital. According to Marx’s analysis of the mere circulation process, surplus value cannot be generated from circulation whether or not there is an equivalent exchange in the circulation process. The four cases analyzed by Marx can be represented by the following “zero-sum game.”

$${\displaystyle \sum }_{i=1}^n{R}_i(S)=0,i.e.,R_i(s_i,s_{-i})=-R_{-i}(s_i,s_{-i})$$

Or, under the mixed strategy:

$${\displaystyle \sum }_{S\in \overline{S}}{R}_i(S)p_i(S)=0,$$

where R is the surplus value ΔG originally defined by Marx, S is the exchange strategy of the commodity owner, and p_i is the probability of choosing a pure strategy. The conclusion that surplus value is the net increase in wealth in a capitalist economy can be reinforced by expressing Marx’s analysis of the contradiction in the general formula for capital using a “zero-sum game.”

Turning labor power into commodities is the social condition for solving the contradiction in the general formula for capital. One of the two basic conditions for turning labor power into commodities is that the owners of labor power have no way of using the means of production P_n and means of consumption P_n to realize their labor power, and as a result, can only sell their labor power A as a commodity, i.e.,

$$\forall A:(P_m=\varnothing ,\mathrm{and}{P}_n=\varnothing )\Rightarrow A=W_A.$$

Applying Marx’s analysis, let the set of commodities necessary for the reproduction of labor power be a bounded set $W_A\subset W^{n+1},$ $W_A=(w_{A,a},w_{A,f},w_{A,e}),$ in which $w_{A,a},w_{A,f},w_{A,e}$ are the commodity equivalents for reproducing the labor power of the workers, the commodity equivalents for sustaining the laborers’ households, and the commodity equivalents for education and training, respectively. The lower bound W_A is the minimum set of commodities $w_A\subset W_A$ that sustains the reproduction of labor power, and the upper bound is determined by the minimum surplus value that decides capital accumulation. Thus, the value of labor power as a commodity is determined by the labor consumption reproducing W_A i.e., $W_A=h(t)f(a),$ or

$$W_A=(t_{a,1},t_{a,2},\dots ,t_{a,n}){(b_{a,1},b_{a,2},\dots ,b_{a,n})}^T=t\mathbf{B},$$

where B is the consumption goods vector necessary for the reproduction of labor power and t is the corresponding time consumption vector. It is obvious that the value of labor power as a commodity depends on labor productivity in the production sector that creates these consumption goods, with which it is negatively correlated. If the labor productivity in sector b_a,i changes, the amount of labor used to produce the same amount of consumption goods and the value of goods will also change. This change affects the value of labor power according to the share of the product in the consumption goods required for the reproduction of labor power. It is of great significance for understanding the principle of relative surplus value production.

Marx emphasized that the currency used to purchase labor power as a commodity performs the function of a means of payment. That is, the workers are not paid the sum $w_A{(A)}_t$ until they have performed $Con{(A;w_A)}_{t-1},$ their function according to the contract $Con(\cdot )$ . Specifically,

$$w_A{(A)}_t=Con{(A;w_A)}_{t-1}.$$

This is what Marx meant by workers granting credit to capitalists. It can be seen from the contradiction inherent in the means of payment that the owners of labor power as a commodity cannot be paid if the capitalist’s commodity is not realized. For this reason, payment for labor power as a commodity is a random event that depends on the realization of the capitalist’s commodity:

$$E{w}_A=s{w}_A+(1-s)(-w_A).$$

The capitalist’s strategy s is to fulfill the contract $Con(\cdot )$ if the commodity can be properly realized, i.e., if $s=1,$ labor power is paid for w_A If the commodity cannot be properly realized, the contract will not be performed, i.e., $s=0,$ and there will be a net loss –w_A for labor power. Marx’s analysis here is in line with reality. At the same time, it sharply contradicts the theory that views workers as risk-averse. In fact, the workers are forced to take risks that have nothing to do with them but are caused by the capitalists.

The nature and characteristics of capitalist production revealed by Marx’s analysis of the process of producing surplus value can be expressed as:

$$\begin{array}{l}\{\begin{array}{l}Q=f(P_m,A)\hfill \\ \{\begin{array}{l}W=H(t)f(A)+C(Q)\hfill \\ W_N=H(t)f(A)\hfill \end{array}\hfill \end{array}\\ \begin{array}{l}\max M\{M|M\in W_C;W_C\subset P_C(X)\}\hfill \\ s.t.\mathbf{f}\in \mathbf{F}\hfill \end{array}\end{array}$$

where $Q=f(P_m,A)$ is the production function, $\mathbf{f}\in \mathbf{F}$ is the feasible production set, and $W_N=V+M$ is the new value. If labor time t_s is used to express value, then $W_N=t_{s}Q=t_{s}f(P_m,A).$ Under normal circumstances, capital purchases labor power $V=W_A$ at value, and the activity of capitalists is to supervise labor $A_C(s_i).$ This activity is not productive labor, i.e., $A_C(s_i)\notin L.$ Therefore, $A_C(s_i){{\displaystyle \cup }}^{}L=\Omega ,$ $A_C(s_i){{\displaystyle \cap }}^{}L=\varnothing ,$ that is, the supervising activities of capitalists are in opposition to productive labor. The capitalist purpose of production can be further expressed as:

$$\max {{\displaystyle \int }}^{}M(Q(L)-V(Q(L)))f(L)dL.$$

Marx’s analysis of labor from the standpoint of the general nature of the labor process is cybernetic in character. As a result, labor can be viewed as a cybernetic process, and using a cybernetic model can be described as:

That is, $y=\frac{S}{1-SR}x,$ where x stands for input, y stands for output, S stands for transmission coefficient of the regulated system, R stands for feedback transmission coefficient, and Δx means feedback input.

In analyzing the labor process and value augmentation process, Marx pointed out that there are quality requirements for both the living and materialized labor that form commodities. In particular, he noted the impact of the quality of intermediate products (inputs) on the quality of subsequent products. This idea can be expressed in a quality-weighted or quality-rated $(\lambda >1)$ model as:

$$Q^{\lambda }=f^{\lambda }(P_m,A).$$

Absolute surplus value production and relative surplus value production constitute surplus value production, and a general significance can be attached to absolute surplus value production. If the length of the working day is t, and the time required for reproducing labor power or the necessary labor time is t_a , then the condition for obtaining surplus value is $(t>t_a)=t_m.$ In other words, the length of the working day must be greater than the time required to reproduce the labor power. This can also be expressed as $t>t_{s}B,$ which is equivalent to Marx’s $(t>t_a)=t_m.$ This is an expression of the general principle of surplus value production. The formula $\frac{t-t_{s}B}{t_{s}B}$ can be used to express the rate of surplus value $m^{\prime }=\frac{m}{v}.$ However, this latter formula needs to be properly understood and applied.

The premise of absolute surplus value production is that the production technology k and the value of labor power as a commodity remain constant $({(k)}^{\prime }=0,{(t_{s}B)}^{\prime }=0),$ so that the surplus value depends on the length of the working day and $m\propto t,$ but $t<24$ (the natural limit of the length of the working day).

Marx’s analysis that the length of the working day is ultimately determined by the balance of power between labor and capital can be expressed as the function:

$$t=f(\sigma ),$$

where $\sigma =S_A/S_C$ is the ratio of the strategy set of workers and capitalists. If the workers are more powerful, then $\sigma >1,$ $\frac{dt}{d\sigma }<0.$ In other words, the working day will be shortened. In the contrary situation, working days will be extended. Marx’s principle that “between equal rights force decides” is of great significance for understanding the specific results of conflict between labor and capital (Marx and Engels 2010, 243).

Marx provided a general mathematical model for the conversion of money into the minimum amount of capital based on his analysis of absolute surplus value. When his analysis is transformed into symbolic language, and the parameters he assumed are generalized, n is the minimum number of workers required to convert money into capital, β is the multiple by which the life of capitalists is better than that of workers, and $\beta \ge 2.$ Meanwhile, α is the proportion of surplus value used by capitalists for accumulation, i.e., the accumulation rate. If the surplus value produced by a single worker is m_i , the minimum number of employed workers required to convert money into capital is:

$$\{\begin{array}{l}n=\frac{\beta }{m^{\prime }(1-\alpha )}\hfill \\ s.t.\beta \ge 2\hfill \end{array}$$

When Marx’s assumptions are plugged in, the result obtained is $n=8.$ The minimum amount of currency required to convert money into capital can easily be obtained from the model. ³

Relative surplus value production is formed spontaneously through competition as individual capital seeks excess surplus value. In Marx’s analysis, it is assumed that the length of the working day remains unchanged $(t^{\prime }=0),$ and that the commodity value per unit of the producer i, determined by social conditions, is:

$$w_{s,i}=\frac{C_{g,i}{T}^{-1}}{Q_i}+C_{l,i}+\frac{n_i{v}_i+M_{s,i}}{Q_i},$$

where $C_{g,i}$ is the fixed capital, T is its service life, $C_{l,i}$ is the current constant capital, and n_i is the number of employed workers. It is assumed that producer i reduces individual labor consumption by improving technology and increasing labor productivity to obtain excess surplus value. According to Marx, an increase in labor productivity is expressed as a diminution of the amount of labor relative to the means of production it drives, that is to say, it is directly reflected in an improved composition of capital and technology. Therefore, let the growth rate of the use of fixed capital be b while the use of workers is reduced at the rate α. Over the same period, the rate of output growth following the increase of labor productivity is g, and $g>b,\alpha <1:$

$$w_i=\frac{C_{g,i}(1+b)T^{-1}}{Q_i(1+g)}+C_{l,i}+\frac{n_i(1-\alpha )v_i+M_{s,i}}{Q_i(1+g)}.$$

From $(1+b)/(1+g)<1$ and $(1-\alpha )/(1+g)<1,$ it follows that $w_i<w_{s,i}.$ At the same time, it also follows that producer i has significantly improved his capital composition on account of technological progress.

$$\frac{C_{g,i}(1+b)+C_{l,i}}{n_i(1-\alpha )v_i}>\frac{C_{g,i}+C_{l,i}}{n_i{v}_i}$$

This is of great significance for understanding the law that the average rate of profit tends to fall. Because producer i that adopts the new technology has a higher output, it is necessary to expand the market. This larger market can be obtained if the producer reduces the commodity price. It is obvious that producer i can obtain a certain amount of excess surplus value provided he sells his commodities at the price $w_i<p_i<w_{s,j}$ (Marx and Engels 2010, 322). If the commodities of producer i are sold at their social value, then from $w_{s,i}{Q}_i(1+g)-C_{g,i}(1+b)T^{-1}-C_{l,i}{Q}_i(1+g)=W_{N,i}>w_{s,i}{Q}_i-C_{g,i}{T}^{-1}-C_{l,i}{Q}_i=W_N$ it follows that $\frac{tnv}{W_{N,i}}=t_{a,i}<\frac{tnv}{W_N}=t_a,$ and thus $t_{m,i}>t_m.$ As long as $w_i<p_i<w_{s,i},$ producer i takes less time to reproduce the labor power than other similar producers. Excess surplus value is also formed by reducing necessary labor time and relatively extending surplus labor time, as Marx pointed out.

The competition among producers for excess surplus value will generalize new technologies which will become a new determinant of socially necessary labor time. With the popularization of new technologies, labor productivity is generally improved, commodity value converges to w_i excess surplus value disappears, and the quantity of value per unit of each commodity drops to the level determined by the new socially necessary labor time. If i belongs to the sector of labor power reproduction, the labor power reproduction time will be reduced according to the proportion of $w_i=t_{s,i}{b}_i$ in tB . When competition increases the general labor productivity in the sector producing B and in those sectors related to it, the time required for labor power reproduction will decrease. If the length of the working day remains unchanged, the surplus labor time is prolonged in relative terms, and the relative surplus value production M_x (L) will eventually be formed.

$$\begin{array}{l}{M}_X(L):L_{b_{ij}}^{\prime },L_{b_{ik}{b}_{kj}}^{\prime },L_{b_{is}{b}_{sk}{b}_{kj}}^{\prime },\dots >L_{b_{ij}},L_{b_{ik}{b}_{kj}},L_{b_{is}{b}_{sk}{b}_{kj}},\dots \\ \frac{t-t_{s}B}{t_{s}B}\to \frac{t-(t_s-\Delta t)B}{(t_s-\Delta t)B}\end{array}$$

The purpose of capitalist production is to maximize the surplus value max M. According to the fundamental theorem of the labor theory of value $\frac{d{W}_A}{d{L}_a}<0$ and the structure of the capitalist working day $t=t_a+t_m,$ it follows that $\frac{d{t}_a}{d{l}_a}<0,$ $\frac{d{t}_m}{d{t}_a}<0,$ and thus $\frac{d{t}_m}{d{l}_a}>0.$ In other words, the amount of commodity value is negatively correlated with labor productivity, while the relative surplus value is positively correlated with labor productivity $\frac{d{M}_X}{dL}>0.$ This is the mathematical form of Marx’s answer to the “riddle with . . . Quesnay” (Marx and Engels 2010, 325).

Marx’s theory of the production of relative surplus value reveals the technical and social conditions of the capitalist labor process, and further discloses the underlying reasons and mechanisms for the continuous changes in the way production is carried on. It thus truly reveals the principles of capitalist innovation, and is obviously superior to Schumpeter’s innovation theory.

If M(T) and M(L) are absolute surplus value production and relative surplus value production, respectively, then the capitalist production set is $M(T){{\displaystyle \cup }}^{}M(L)=\Omega$ , and $M(T){{\displaystyle \cap }}^{}M(L)\ne \varnothing .$

Combining the theory of labor as a commodity and the theory of capital accumulation, Marx’s wage $(\omega )$ determination theory can be expressed by the functional equation as:

$$\omega =f(W_A,\Delta C),and\frac{d\omega }{d{W}_A}>0,\frac{d\omega }{d\Delta C}<0,$$

where ΔC denotes capital accumulation, or

$$\omega =f(W_A,A^s(\Delta C)),\frac{d\omega }{d{W}_A}>0,\frac{d\omega }{d{A}^s}<0,\frac{d{A}^s}{d\Delta C}>0,$$

where A^s is labor supply. Wages conceal the division of workers’ labor into necessary labor and surplus labor. The hourly wage $\omega t$ is only the payment for paid labor, but it takes the form of payment for all labor. Similarly, if $t>t_{s}B,$ and if $\alpha =\frac{1}{t}$ is the α unit of means of consumption per hour obtained by workers who provide one unit of labor power per hour, then $\alpha t_{s}B$ is only the payment for paid labor but takes the form of the payment for all labor (Zhang and Xue 2020).

The Formalized Outline of Capital Accumulation Theory

Marx’s analysis of capital accumulation begins with capitalist simple reproduction. Through his analysis of simple reproduction under capitalism, he revealed three new characteristics of the capitalist production process. These can be expressed as follows.

First, the variable capital prepaid by capitalists in the form of wages is created by workers’ own labor. That is, the capitalists use the products produced by the workers and realized in period $t-1$ to pay the workers employed in period $t-1$ only in period t:

$${\omega }_t=v^{\prime }h(t)f{(a)}_{t-1},$$

where $v^{\prime }=\frac{v}{w_N}$ is the share received by workers in the new value they create, that is, the proportion of paid labor in the total new value. Incidentally, it is inconsistent with Marx’s theory of wages that the economy in which wages are paid at the beginning of the capitalist production cycle is described by Morishima as the “Marx-Von Neumann economy” (Morishima 2017, 128, 13 Footnote 1). In reality, the currency that pays wages performs the function of the means of payment, and is paid at the end of the period rather than at its beginning.

Second, under the conditions of simple reproduction the original prepaid capital will be transformed into capitalized surplus value after a certain production time, i.e.,

$$C_0=\underset{0}{\overset{\tau }{{\displaystyle \int }}}m(t)dt,$$

where C ₀ is the original prepaid capital, and the process is assumed to be continuous.

Third, workers’ individual consumption is subordinate to capital. Thus, the reproduction process is at the same time the reproduction of a capitalist production relation which is equivalent to a fixed point, that is,

$$f(P_C)=P_C,$$

where $P_C=P(C,V;m)$ is the capitalist production relation.

Through his analysis of capital accumulation, Marx revealed how the law of commodity production ownership became transformed into the law of capitalist possession. According to Marx’s analysis, capital accumulation is the process through which capitalists transform (⇒) uncompensated value appropriation into capital.

$$(1-v^{\prime })h(t)f(a)=m\Rightarrow (\Delta c+\Delta v)$$

If it is assumed that the original prepaid capital is ${(c+v)}_0,$ then the process of capital accumulation can be expressed as:

$$\begin{array}{l}{(c+v)}_0\mathrm{output}{m}_0\Rightarrow {(\Delta c+\Delta v)}_1\\ {(c+v)}_0+{(\Delta c+\Delta v)}_1\mathrm{output}{m}_0+\Delta m_1\Rightarrow {(\Delta c+\Delta v)}_1+{(\Delta c+\Delta v)}_2,\end{array}$$

that is,

$${(c+v)}_0+{\displaystyle \sum }_{t=1}^n{(\Delta c+\Delta v)}_t\mathrm{output}{m}_0+{\displaystyle \sum }_{t=1}^n\Delta m_t\Rightarrow {\displaystyle \sum }_{t=1}^{n+1}{(\Delta c+\Delta v)}_t.$$

This expresses what Marx pointed out: the additional capital is capitalized surplus value, and is produced by the unpaid labor of others. The working class has always created the capital that in coming years will employ additional labor by using the surplus value produced this year, that is, by “creating capital out of capital.” The capitalists’ past ownership of unpaid labor is the only condition needed for them to utilize living unpaid labor on an ever-increasing scale, and the more the capitalists have accumulated, the more they can accumulate.

It is evident from the process of capital accumulation that the additional capital that its owners exchange for labor is a portion of the product of other people’s labor that has been appropriated without paying the equivalent, although capital buys labor at the value $\Delta v=\Delta w_A=\Delta t_{s}B.$ This part of the capital must be compensated not only by the workers who produce it, but also by adding a new surplus to the compensation, i.e., $w_N=h(t)f(a)=\Delta v+\Delta m.$ For this reason, exchange becomes a superficial phenomenon. The situation shows that the law of ownership of commodity production has been transformed into the law of capitalist possession. The key to this transformation is that labor power becomes the worker’s own commodity:

$$E(q)=\{A|A=W_A\subset W^{n+1};W_A=t_{s}B\}.$$

Workers only have the right to claim the value of their labor power as a commodity, that is, the right to ask for equivalent exchange $W_A=t_{s}B.$ Once labor power is sold in the production process, labor will be subordinate to capital and will create surplus value for capitalists under their supervision.

$$(1-v^{\prime })h(t)f(a)=m\subset P_C(X)$$

Ownership $P(W_N)$ is separated from labor L_A :

$$L_A{{\displaystyle \cap }}^{}P(W_N)=\varnothing .$$

Surplus value becomes wealth $P_C(X)$ owned by capitalists, rather than the wealth of the workers who are its direct producers. It is not against the law of value that capitalists accumulate using the surplus value belonging to them, but the mode of possession has changed fundamentally.

With the accumulation of capital, the original prepaid capital is dimensionless compared to the direct capital accumulation.

$$\underset{t\to n}{\lim }\frac{C_0}{{\displaystyle \sum }_{t=1}^n\Delta C_t}=0$$

Marx analyzed the determinants of the amount of capital accumulation by first analyzing the division of surplus value into capital (accumulation $z=\Delta c+\Delta v)$ and income (capitalists’ income y for consumption) under the condition that the amount of surplus value was certain, as determined by the capital accumulation rate. The capital accumulation rate can be defined as:

$$\alpha =\frac{\Delta c+\Delta v}{m}=\frac{z}{m},0\le \alpha \le 1or\alpha \in (1,0),$$

$m=z+y,z\propto y^{-1},\mathrm{or}m=p_c{q}_c+p_y{q}_y.$ The accumulation rate is determined by the individual capitalist, but not by the so-called “abstinence” behavior of the individual capitalist. Instead, the capitalist is performing the functions of capital under the surplus value motive and competitive pressure. However, it can be seen from $y_t=\alpha m_{t-1}{\pi }^{\prime }(1-\alpha )$ and ${\pi }^{\prime }=\frac{m}{c+v}$ that the capitalist’s consumption increases with accumulation, and there is no conflict between the two.

$$y_0<y_0+y_1<y_0+y_1+y_2<\dots$$

It is obvious that the amount capitalists consume depends on the surplus value they acquire. At the same time, and as Marx pointed out, spendthrift consumption is a means for capitalists to obtain credit, and has thus become a business necessity. That is to say, spendthrift consumption is a special signal used by capitalists to show their creditworthiness. If the mortgaged wealth of capitalists $\theta =({\theta }_1,\dots ,{\theta }_n)$ is private information, the type $\rho$ of capitalists will depend on $\theta .$ However, regardless of other circumstances, the consumption type of capitalists $x\in X$ is observable common knowledge. Meanwhile, it is assumed that there is a linear relationship between the type of consumption practiced by capitalists and their wealth:

$$x_i^{\rho }=c_0+c_1{y}_i.$$

Spendthrift consumption by individual capitalist i will be matched with mortgaged wealth θ with probability ϕ. In this way, spendthrift consumption becomes a signal showing that a capitalist is likely to be able to repay credit, and is an important means for the capitalist to obtain further loans.

At a certain ratio (α) of surplus value in relation to capital and income, capital accumulation depends on the absolute amount of surplus value, and the cases that determine the amount of surplus value will affect the capital accumulation. Marx analyzed four cases or factors that determine capital accumulation, and that can be expressed as accumulation functions:

$$Z=\Delta C=f(E(\cdot ),L_s(\cdot ),D,C),\mathrm{and}\frac{dZ}{df}>0.$$

The first of these factors is an increase in the level of labor exploitation, i.e.,

$$E=f(v_c,t,L_q),\mathrm{and}\frac{dE}{df}>0.$$

This includes forcing wages below the value of labor power $\omega =t_{s}B-v_c,$ extending the working day t and increasing labor intensity L_q . Lowering wages serves to convert part of the worker’s consumption fund into accumulation fund v_c A common means of lowering wages is to degrade daily consumption materials, namely, $B^{\lambda }(\lambda <1).$ Extending working days and increasing labor intensity does not increase fixed constant capital C_g , nor does it raise variable capital in proportion. Meanwhile, these measures increase the surplus value for accumulation Δm:

$$c_g+c_l+\Delta c_l+v+\Delta v+m+\Delta m$$

Marx defined the result of extending working days and increasing labor intensity as the expansion ability of capital, that is,

$$\begin{array}{l}(c+v)\to m\\ {(c+v)}^{\beta }\to m+\Delta m,\beta >1.\end{array}$$

The latter is the expansion capacity of capital, i.e., ${(c+v)}^{\beta }>(c+v).$

The second factor is the improvement of social labor productivity, that is,

$$\frac{dQ}{dt}>0and\frac{dW}{dL}<0\to \frac{dZ}{dQ}>0or\frac{dZ}{dL}>0.$$

According to Marx’s analysis, the opposite movement between the magnitude of value and the magnitude of use value that results from the improvement of labor productivity generates an income effect and a substitution effect:

$$w_{i,t+1}-w_{i,t}=-r;w_{ij}^{ji}.$$

The role of these two effects in capital accumulation forms the capital accumulation effect. According to Marx, these effects are manifested in three aspects. The first is the scale effect of capital accumulation.

If the accumulation rate is constant $\frac{d\alpha }{dt}=0,$ the consumption b_c and accumulation by capitalists represented by the vectors will increase simultaneously:

$$b_{C,t+1}>b_{C,t},z_{t+1}>z_t.$$

If capitalists keep their real consumption unchanged $B_{C,t+1}=B_{C,t},$ the accumulation rate can increase $\frac{d\alpha }{dt}>0,$ and accumulation then increases $z_{t+1}>z_t.$

As the price of the means of consumption decreases $p_{II,t+1}<p_{\mathrm{II},t},$ then if the real consumption of workers remains unchanged $B_{t+1}=B_t,$ then $v_{t+1}=v_t,$ whereas $A_{t+1}>A_t.$ That is to say, the capitalist can hire more workers with the same amount of variable capital.

As the price of the means of production decreases $p_{\mathrm{I},t+1}<p_{\mathrm{I},t},$ then $C_{t+1}=C_t,$ whereas $P_{m,t+1}>P_{m,t}.$

The second effect is the efficiency effect of capital accumulation.

For fixed constant capital, there is

$$C_{g,R}^a,a>1,C(1-\beta )+C_R^a>C(1-\beta )+\beta C,$$

where $\beta =T^{-1}$ is the depreciation rate, $C_R^a$ is the partial renewal, and the depreciation expense can be expressed as $C_R^a=\beta C=D.$

For current constant capital, there are

$$\begin{array}{l}{C}_{l,t+1}^a>C_{l,t},a>1\\ C+V=K,K_{t+1}^a>K_t,a>1.\end{array}$$

The last effect is the old value transfer effect of capital accumulation. The value of the means of production can be maximally transferred to new products: $C^a{T}^{-1}>C{T}^{-1}(1-\beta ),a>1,\beta >0$ (waste coefficient).

The third factor is the widening gap between capital employed and capital expended. If K is the investment at the annual rate r, C is the total capital or the total capital employed, $T\ge \tau$ is the capital service cycle, R is the scale of fixed capital renewal, D is the depreciation or the capital employed, and I_D is the depreciation investment rate, then

$$\begin{array}{l}{R}_t=K_{t-\tau }=e^{r(t-\tau )}\\ C={\displaystyle {\int }_{t-\tau }^{t}Kdt=\frac{e^{rt}(1-e^{-r\tau })}{r}}\\ D=\frac{C}{\tau }=\frac{e^{rt}(1-e^{-r\tau })}{r\tau }\\ I_D=1-\frac{R}{D}=1-\frac{r\tau }{e^{r\tau }-1}.\end{array}$$

The widening gap between the capital employed and the capital expended affects capital accumulation in two ways. On the one hand, services can be provided at no cost by the part of fixed capital equivalent to D (the value has been transferred) when the fixed constant capital has worked on the whole. On the other hand, the depreciation fund D can be directly used for accumulation to expand the scale of capital, and $K_D=D{I}_D=D-R$ is the scale of accumulation with the depreciation fund. ⁴

The fourth factor is the increase in the amount of prepaid capital. If $\frac{d{m}^{\prime }}{dt}=0,$ then from $f:V\to M,g:M\to Z$ it follows that

$$\begin{array}{l}\frac{dM}{dV}>0,\frac{dV}{dC}>0;\frac{dZ}{dM}>0\\ n{f}_K\succ f_K\subset F,\end{array}$$

where f_K is the scale of prepayment capital expressed by the production function. It is obvious that the capital of scale nf_K is superior to that of scale f_K Capitalists are able not only to enjoy extravagance but also to accelerate capital accumulation because the surplus value increases as the scale of prepayment capital expands. At the same time, the more the scale of production increases along with the amount of prepaid capital, the larger the scale of capital becomes, and the better it is able to take full advantage of the various factors that accelerate accumulation.

Marx analyzed the impact of capital accumulation on the fate of the working class from the two standpoints of a constant organic composition of capital and an increased organic composition of capital. His reasoning can be formally restated as follows.

If the organic composition of capital is constant $\frac{dk}{dt}=0,$ it will follow from $z=\Delta c+\Delta v$ that the demand for labor is $v+\Delta v=A^d.$ There will be two cases of capital accumulation under such conditions.

In the first case, when $\frac{dz}{dt}>0$ and $\frac{d^{2}z}{d{t}^2}>0,$ it follows that $\frac{dv}{dt}>0$ and $\frac{d^{2}v}{d{t}^2}>0.$ Thus $A^d>A^s\to \frac{d\omega }{dt}>0,\frac{d(\frac{A^u}{A})}{dt}<0(A^u$ is the unemployed population, A^s is the labor supply). In other words, it is not the absolute or relative increase of the labor force or of the worker population that causes the surplus of capital. On the contrary, it is the growth of capital that causes the shortage of labor available for exploitation.

In the second case, if $\frac{d\omega }{dt}>0\to \frac{dm}{dt}<0\mathrm{and}\frac{d^{2}m}{d{t}^2}<0,\mathrm{then}\frac{dz}{dt}<0,$ thereby $\frac{dv}{dt}<0\mathrm{and}\frac{d^{2}v}{d{t}^2}<0,\mathrm{then}{A}^s>A^d,\frac{d\omega }{dt}<0,\frac{d(\frac{A^u}{A})}{dt}>0.$ In other words, it is not the absolute or relative increase of the labor force or the worker population that causes the shortage of capital. On the contrary, it is the reduction of capital that leaves a surplus of labor available for exploitation or the overpricing of labor power.

It is obvious that $\frac{d\omega }{dt}<0$ will restore surplus value $\frac{dm}{dt}>0,$ so that capital accumulation will resume $\frac{dz}{dt}>0.$ With the increase in accumulation, the two cases will be alternated. In other words, there will be a periodic relative surplus population unrelated to the natural population. This also demonstrates the instability of capitalist employment and workers’ incomes.

Thus, accumulation is the independent variable and wages are the dependent variable $\omega =f(z)$ :

$$\begin{array}{l}\frac{d\omega }{dt}>0\mathrm{or}\frac{dz}{dt}>0,\mathrm{if}\mathrm{and}\mathrm{only}\mathrm{if}\frac{dz}{dt}>0\mathrm{and}\frac{d^{2}z}{d{t}^2}>0,A^d>A^s.\\ \frac{d\omega }{dt}<0\mathrm{or}\frac{dz}{dt}<0,\mathrm{if}\mathrm{and}\mathrm{only}\mathrm{if}\frac{d\omega }{dt}>0,\frac{dm}{dt}<0\mathrm{and}\frac{d^{2}m}{d{t}^2}<0,\frac{dz}{dt}<0,A^s>A^d.\end{array}$$

However, on the surface, wage changes are expressed as a result of changes in labor supply, which in turn are expressed as a result of absolute increases or decreases in population (P), i.e.,

$$\omega =f(A^s(P))\mathrm{and}\frac{d\omega }{dP}<0,P(t)=P_0{e}^{pt}\cap Z=\varnothing .$$

This is the so-called “law of natural population.”

To sum up, the law of capitalist accumulation revealed by Marx (Marx and Engels 2010, 614–616) can be described as:

$$\{\begin{array}{l}\omega =f(z)\\ s.t.\max \omega \le \omega (z_{\min })\\ \min E_A\ge E_A(z_{\min })\end{array}$$

where E_A is the degree of labor exploitation.

In the case of an increase in the organic composition of capital $\left(\frac{dk}{dt}>0\right)$ , capital accumulation will lead to a relative overpopulation P_X . The increase in the organic composition of capital is the product of capital accumulation leveraged by the development of social labor productivity, i.e.,

$$k=f(z(L_s)),\frac{dk}{d{L}_s}=\frac{dk}{dz}\cdot \frac{dz}{d{L}_s}>0,z=f(L_s),L_s=g(z).$$

The interaction between capital accumulation and improved labor productivity increases the scale of individual capital and the organic composition of capital. The increase in individual capital includes capital accumulation and capital concentration. The capital concentration that is favorable to capital accumulation can be expressed as:

$$(1-v^{\prime })h(t)f(a)=m\to (\Delta c+\Delta v).$$

Capital centralization can be expressed as:

$$f_1,\dots ,f_n\to F(x_1,\dots ,x_n)andF(x_1,\dots ,x_n)>{\displaystyle \sum }_{i=1}^n{f}_i(x),$$

where x_i is the factor of production. One of the important factors contributing to capital centralization is the cost advantage enjoyed by large-scale capital when it seeks to compete on the basis of low price:

$$K_F<K_{-F}or\frac{C_{g,i}(1+b)T^{-1}}{Q_{F,i}(1+g)}<\frac{C_{g,i}{T}^{-1}}{Q_{-F,j}}.$$

Thus, the price $p_F<p_{-F}$ . The increase in capital scale will improve the organic composition of capital.

The demand of capital for labor depends on variable capital $A^d=f(V)$ , but $\frac{dV}{dZ}<0,$ thus $\frac{dZ}{d{A}^d}<0.$ It is assumed that population remains constant $\frac{dP}{dt}=0;$ the unemployed population A ^u will then increase with capital accumulation and stabilize at a level appropriate to the needs of capitalism:

$$\frac{dZ}{d{A}^u}>0,\mathrm{when}{Z}_{\max },A^d(t)=A^s(t),\exists A^u=P_X.$$

Relative overpopulation is the product of capital accumulation, and therefore, it can be explained from the rate of change of unemployment that a relative overpopulation is the normal state of a capitalist economy. It is assumed that if P is the natural population and remains constant $\frac{dP}{dt}=0,$ while a is the constant labor force rate, then the labor population will be P_a = aP. Meanwhile, if it is assumed that a_u is the unemployment rate, λ_u is the probability of unemployment, and λ_e is the probability of employment, then the number of newly added unemployed people will be ${\lambda }_u(1-a^u)P_a,$ the number of re-employed people in the unemployed population will be ${\lambda }_e{a}^u{P}_a,$ and the rate of change of unemployment can be expressed as:

$$\begin{array}{l}\frac{d(a^u{P}_a)}{dt}={\lambda }_u(1-a^u)P_a-{\lambda }_e{a}^u{P}_a,0<{\lambda }_e<1,0<{\lambda }_u<1\\ \frac{d{a}^u}{dt}={\lambda }_u(1-a^u)-{\lambda }_e{a}^{u}or\frac{d{a}^u}{dt}={\lambda }_u-({\lambda }_u+{\lambda }_e)a^u.\end{array}$$

Solving the differential equation $\frac{d{a}_u}{dt},$ the long-term unemployment rate can be obtained as:

$$a^u(t)=\frac{{\lambda }_u}{{\lambda }_u+{\lambda }_e}+(a_0^u-a_t^u)e^{-({\lambda }_u+{\lambda }_e)t}.$$

Obviously, $\underset{t\to \infty }{\lim }(a_0^u-a_t^u)e^{-({\lambda }_u+{\lambda }_e)t}=0.$ The unemployment rate converges to the steady state $a^u=\frac{{\lambda }_u}{{\lambda }_u+{\lambda }_e},$ the long-term steady-state unemployment population is $a^u{P}_a,$ and the steady-state unemployment rate is $a^u=\frac{{\lambda }_u}{{\lambda }_u+{\lambda }_e}.$ Replacing $P_a$ with $P_a=aP,$ the above conclusion remains unchanged.

It follows that the unemployed population under capitalism is unrelated to the natural population and its growth rate, but is the relative overpopulation determined by capital accumulation.

From Marx’s analysis of the primitive accumulation of capital, it is clear that the capitalist mode of production is based on the violent deprivation $(R_F)$ of small production. Taking into account the spontaneous differentiation of producers, the following deprivation process functions can be established:

$$\begin{array}{l}{N}_b=N_b(t_a,R_F)\\ d{N}_b=\frac{\partial N_b}{\partial t_a}d{t}_a+\frac{\partial N_b}{\partial R_F}d{R}_F,\\ \mathrm{and}\frac{\partial N_b}{\partial R_F}d{R}_F>\frac{\partial N_b}{\partial t_a}d{t}_a,\mathrm{where}{t}_a>t_s,\frac{d{N}_b}{d{R}_F}>0,\frac{d^2{N}_b}{d{R}_F^2}>0.\end{array}$$

Or

$$\begin{array}{l}\frac{d{N}_b}{N_b}=\frac{\partial N_b}{\partial t_a}\cdot \frac{t_a}{N_b}\cdot \frac{1}{t_a}d{t}_a+\frac{\partial N_b}{\partial R_F}\cdot \frac{R_F}{N_b}\cdot \frac{1}{R_F}d{R}_F\\ \frac{\partial N_b}{\partial t_a}\cdot \frac{t_a}{N_b}=\frac{\partial N_b/\partial t_a}{N_b/t_a}=\delta ,\frac{\partial N_b}{\partial R_F}\cdot \frac{R_F}{N_b}=\frac{\partial N_b/\partial R_F}{N_b/R_F}=\epsilon \\ \frac{d{N}_b}{N_b}=\delta \frac{d{t}_a}{t_a}+\epsilon \frac{d{R}_F}{R_F}.\end{array}$$

From $P(t_a)P(\overline{b}|t_a)$ and $P(t_a)(b|t_a),$ it follows that $\epsilon >>\delta .$ This shows that organized violence $(R_F)$ accelerates the process through which workers are deprived of their means of production, that is,

$$L_A\cap P(P_m)=\varnothing .$$

The formation of the class of wage workers is thus accelerated.

The Premise and Limit of Formalization of Marx’s Economics

In the above, we have tried to visualize the second case of Marx’s mathematical methods, taking the first volume of Capital as an example. It should be emphasized in particular that the formalization of Marx’s economics must accurately reflect the principles of his economics, or else serious problems will arise. For instance, Michio Morishima, ⁵ a famous Japanese mathematical economist and representative of the School of Marxian Mathematical Economics, argued that Marx had two definitions of value that differ in insight. One definition states: “All that these things now tell us is, that human labour power has been expended in their production, that human labour is embodied in them. When looked at as crystals of this social substance, common to them all, they are – Values” (Marx and Engels 2010, 48). The other is: “We see then that that which determines the magnitude of the value of any article is the amount of labour socially necessary, or the labour time socially necessary for its production” (Marx and Engels 2010, 49). Taking a society that produces grain a ₁₁ and fertilizer a ₂₁ as an example, Morishima argued that Marx’s former value was defined by the amount of labor consumed directly l ₁ and the amount of labor consumed indirectly $a_{11}{\lambda }_1+a_{21}{\lambda }_2$ in producing 1 unit of a commodity. The latter amount is defined by the total quantity of labor consumed ( $l_1{q}_1+l_2{q}_2$ , i unit of total output of product q_i ) by various units of the total output that needs to be invested to produce one unit of net output. In other words, value is defined according to the input–output relationship. Thus, these two definitions of value are completely different in their mathematical expressions; one is ${\lambda }_1=a_{11}{\lambda }_1+a_{21}{\lambda }_2+l_1$ and the other is ${\mu }_1=l_1{q}_1+l_2{q}_2,$ where λ ₁ and µ ₁ are both the value of grain (Morishima 2017, 12). It is clear that Morishima confused Marx’s qualitative and quantitative provisions concerning value. The value determination equation formulated by Morishima, based on the input–output model, reflects neither Marx’s concept of socially necessary labor time nor Marx’s conclusions concerning the social conditions that determine socially necessary labor time. Instead, it rests on the total amount of labor consumed to produce a unit of net product under the multiplier effect. Morishima argued, on the one hand, that Marx’s two definitions of value represent different insights into value, while, on the other hand, seeking to prove by mathematical derivation that these two definitions are perfectly equivalent (Morishima 2017, 18). As a result, Morishima’s thinking contains an obvious logical contradiction.

Morishima saw the core of Marx’s economics as a general equilibrium model containing value theory and reproduction theory. Accordingly, Morishima argued that value theory itself could not explain consumer demand, and that there would not be general equilibrium without some kind of consumer demand theory. For this reason, and referring to Marx’s discussion of commodity attributes, Morishima contended that Marx would have accepted the theory of the marginal utility of consumer demand if it had been known to him (Morishima 2017, 18). ⁶ According to Morishima’s understanding and interpretation of Marx’s exposition, however, it follows necessarily that $\frac{u_A}{P_A}=\frac{u_B}{P_B}=\frac{u_1}{P_1}=\frac{u_2}{P_2}=\dots =\frac{u_m}{p_m}=v,$ and then $u_i=v{p}_i$ . In other words, marginal utility is equal to commodity value. Obviously, Marx could not have accepted this theory. Morishima regarded Marx’s economics as a general equilibrium model, which is the product of a misreading that perverts Marx’s economics.

As interpreted by Morishima, Marx’s economics has a dual system of calculating values and prices, that are not uniform in dimension and cannot be compared. Attempting to unify these dimensions, Morishima measured commodity prices using the wage rate w, that is, $p_{i,w}=\frac{p_i}{w}.$ . He believed that in this way price $p_{i,w}$ and value λ_i could be measured by labor. Morishima applied this method to study the relationship between commodity value and the price calculated by the labor wage rate in simple commodity production and capitalist production. In simple commodity production:

$$\begin{array}{l}{p}_i=p_j{a}_{ji}+w{l}_i,\\ p_{i,w}=p_{j,w}{a}_{ji}+l_i.\end{array}$$

Thus, $p_{i,w}={\lambda }_i,$ in which the price of a commodity calculated on the basis of labor is equal to the value of the commodity.

In a capitalist economy, workers are exploited by capitalists. When there is positive profit in each industry, it will follow that

$$p_i>p_j{a}_{ji}+w{l}_i.$$

The price of a commodity calculated on the basis of labor will be greater than its value:

$$p_{i,w}>p_{j,w}{a}_{ji}+l_i.$$

In the case of the production price, it will follow that

$$\begin{array}{l}{p}_i=(1+\pi )(p_j{a}_{ji}+w{l}_i),\\ p_{i,w}=(1+\pi )(p_{j,w}{a}_{ji}+l_i).\end{array}$$

Obviously, the price calculated on the basis of labor, i.e., the wage rate, makes the sum of the production prices of commodities greater than the sum of their values, the cost price of a commodity greater than its value, and the total profit greater than the total surplus value (Morishima 2017, 79–80). This is a complete deviation from Marx’s economics. The fundamental reason why Morishima had such a serious problem is that he did not understand the essence of capitalist wages. While wl_i is the payment for necessary labor at the monetary wage rate, it takes the form of payment for all living labor l_i . Thus, the fact that workers’ labor is divided into necessary labor and surplus labor is completely concealed. Calculating the price of a commodity using the wage rate, that is, transforming p_i to $p_{i,w}$ , is equivalent in the terms of pure mathematics. In economics, however, the cost consumption or cost price of a commodity $p_j{a}_{ji}+w{l}_i$ becomes the value of the commodity $p_{j,w}{a}_{ji}+l_i$ after adjustment is made for the consumption of constant capital at the prevailing wage rate. This represents a fundamental change in the nature and meaning of the formula, and failure to notice this change will inevitably lead to completely erroneous conclusions. At the same time, the price is converted into the labor time of workers calculated using the wage rate as the calculation unit. What this in fact determines is the commodity value in terms of the labor purchased, instead of the labor consumed in production. This is Smith’s erroneous value theory, not Marx’s value theory. Here Morishima inherited the error of Nobuo Okishio (Okishio 2010; Zhang and Xue 2020). It was on the basis of this erroneous treatment and its results that Samuelson rejected Marx’s labor theory of value, and in particular, his theory of surplus value (Samuelson 1957).

It is evident that in formalizing Marx’s economics using mathematical methods, one must strictly follow the basic principles of Marx’s economics. The premise and limit of formalizing Marx’s economics is that economic logic must not be replaced with mathematical logic.

Acknowledgements

This article was translated by Associate Professor Yexia Sun at the School of Marxism, Northeast Normal University, China.

Notes

1.

The symbols w and W in the text denote commodities, sets of commodities, commodity space, total volume of commodities, commodity value, and total commodity value. Many symbols are used in this article, and they should be read according to the definitions given in the text. In addition to the symbols defined by Marx, it is necessary to add some symbols in line with Marx’s discussion. This may result in different meanings being implied by the same symbol.

2.

For a detailed discussion, see Zhang (2011).

3.

Marx’s model is rigorous and general, not provided arbitrarily.

4.

Domar (1983) is referred to in the mathematical model of the third factor.

5.

See Zhang (2018) for a critique of the main problems in formalizing Marxist economics with the mathematical methods advanced by Morishima.

6.

Contrary to Morishima’s speculation, Marx took a negative view of the marginal utility theory.

References

1. E. Domar 1983. The Theory of Economic Growth. [In Chinese.] Translated by J. Guo. Beijing: The Commercial Press.

2. K. MarxF. Engels. 2010. Marx and Engels Collected Works, vol. 35. London: Lawrence & Wishart Electric Book.

3. M. Morishima 2017. Marx’s Economics: A Dual Theory of Value and Growth. [In Chinese.] Beijing: China Social Sciences Press.

4. N. Okishio 2010. “Technological Change and Profit Rate.” [In Chinese.] Teaching and Research, no. 7: 48–56.

5. P. A. Samuelson 1957. “Wages and Interest: A Modern Dissection of Marxian Economic Models.” The American Economic Review 47 (6): 884–912.

6. X. Zhang 2009. “Reflections on the Application of Mathematics in Political Economy.” [In Chinese.] Teaching and Research, no. 9: 33–38.

7. X. Zhang 2011. “Reflections on the Relationship between Labor Productivity and Commodity Value.” [In Chinese.] Teaching and Research, no. 7: 54–59.

8. X. Zhang 2018. “A Critical Review of Morishima’s Formalized Marxian Economics.” [In Chinese.] Studies on Marxism, no. 11: 76–87, 164.

9. X. ZhangY. Xue. 2020. “A Critical Deconstruction of the Okishio Theorem.” World Review of Political Economy 13 (2): 154–191.